Benutzer:Dirk Huenniger/Mathe

Aus Wikibooks

Mathematik Nachhilfe von Linda.

Inhaltsverzeichnis

1 Das griechische Alphabet
2 Mathematische Symbole
3 Begriffe aus der allgemeinen Mathematik
4 Bruchrechnung
5 Umrechnen von Einheiten
6 Geometrie
7 Prozentrechnung
8 Zinsrechnung
9 Zinseszinz
10 Lineare Funktionen
- 10.1 Schnittpunkt
11 Lösungverfahren
12 Quadratische Funktionen
13 Natürliche Zahlen
14 Schriftliche Addition
15 Schriftliche Subtraktion
16 Schriftliche Multiplikation
17 Schriftliche Division

[Bearbeiten] Das griechische Alphabet

Großbuchstabe	Kleinbuchstabe	Name
$A$	$α$	Alpha
$B$	$β$	Beta
$Γ$	$γ$	Gamma
$Δ$	$δ$	Delta
$E$	$ε$	Epsilon
$Z$	$ζ$	Zeta
$H$	$η$	Eta
$Θ$	$\vartheta$	Theta
$I$	$ι$	Iota
$K$	$κ$	Kappa
$Λ$	$λ$	Lambda
$M$	$μ$	My
$N$	$ν$	Ny
$Ξ$	$ξ$	Xi
$O$	$o$	Omikron
$Π$	$π$	Pi
$P$	$ρ$	Rho
$Σ$	$σ$	Sigma
$T$	$τ$	Tau
$>$	$υ$	Ypsilon
$Φ$	$φ$	Phi
$X$	$χ$	Chi
$Ψ$	$ψ$	Psi
$Ω$	$ω$	Omega

[Bearbeiten] Mathematische Symbole

[Bearbeiten] Mengenlehre

Symbol	Bedeutung
$\in$	ein Element von
$\notin$	kein Element von
$\subset$	Teilmenge von
$\subseteqq$	Teilmenge oder gleiche Menge
$\cup$	vereinigt mit
$\bigcup_{i=1}^{n}$	Vereinigung von Mengen
$\cap$	geschnitten mit
$\bigcap_{i=1}^{n}$	Schnitt von Mengen
$\setminus$	ohne bzw. Differenzmenge
$\varnothing$	leere Menge
${}$	leere Menge
$\mathbb{L}$	Lösungsmenge
$\mathbb{G}$	Grundmenge
$\mathbb{N}$	Menge der natürlichen Zahlen ${1,2,...}$
$\mathbb{N}_0$	Menge der natürliche Zahlen inklusive Null ${0,1,2,...}$
$\mathbb{Z}$	Menge der ganzen Zahlen ${..., - 2, - 1,0,1,2,...}$
$\mathbb{Q}$	Menge rationalen Zahlen, bzw. Menge der Brüche
$\mathbb{R}$	Menge der reellen Zahlen
$\mathbb{C}$	Menge der komplexen Zahlen

[Bearbeiten] Algebra

Symbol	Bedeutung
$=$	gleich
$\neq$	ungleich
$: =$	definiert als
<	kleiner als
>	größer als
$\leq$	kleiner gleich
$\geq$	größer gleich
$+$	plus, Additionszeichen
$-$	minus, Subtraktionszeichen
$\cdot$	mal, Multiplikationszeichen
$:$	geteilt, Divisionszeichen
$Σ$	Summe
$Π$	Produkt
$a n$	Potenz (a=Basis, b=Exponent)
$\sqrt{\text{ }}$	Wurzel
$\sqrt[n]{\text{ }}$	n-te Wurzel
$log b$	Logrtithmus zur Basis b
$ln$	natürlicher Logarithmus (zur Basis $e$ )
$lg$	10er Logarithmus (zur Basis 10)
$ld$	dyadischer Logarithmus (zur Basis 2)
$!$	Fakultät ( $4!=4 \cdot 3 \cdot 2 \cdot 1 = 24$ )
$\left( \begin{matrix} n \\ k \end{matrix}\right)$	Binomialkoeffizient $\begin{matrix} \frac{n!}{\left(n-k\right)! k!} \end{matrix}$ z.B. $\left( \begin{matrix} 8\\ 5 \end{matrix}\right)=\frac{8\cdot 7\cdot 6}{5 \cdot 4 \cdot 3 \cdot 2 \cdot 1}$
$\| a \|$	Betrag von a
$i$	imaginäre Einheit
$j$	imaginäre Einheit (in der Elektrotechnik)
$\overline{a}$	konjugiert komplexe Zahl zu $a$
$\overrightarrow{a}\odot\overrightarrow{b}$	skalares Produkt der Vektoren $\overrightarrow{a}$ und $\overrightarrow{b}$ z.B. $\left( \begin{matrix} 2 \\ 1 \end{matrix}\right) \odot \left( \begin{matrix} 3 \\ 4 \end{matrix}\right)= 2 \cdot 3 + 1 \cdot 4 = 10$
$\overrightarrow{a}\times\overrightarrow{b}$	vektorielles Produkt der Vektoren $\overrightarrow{a}$ und $\overrightarrow{b}$ $\left\|\overrightarrow{a}\times\overrightarrow{b}\right\|=\left\|\overrightarrow{a}\right\| \cdot \left\|\overrightarrow{b}\right\| \sin \left(\angle \left(\overrightarrow{a},\overrightarrow{b} \right)\right)$
$\left\|\overrightarrow{a}\right\|$	Länge des Vektors $\overrightarrow{a}$ z.B. $\left\|\left( \begin{matrix} 3\\ 4\end{matrix}\right)\right\|=\sqrt{3^2+4^2}=\sqrt{25}=5$
$\mid$	ist Teiler von z.B. $3 \| 15$ (3 ist Teiler von 15)
$\nmid$	kein Teiler von
$\left( a_{ik} \right)$	Matrix mit den Elementen $a i k$
$\left\| a_{ik} \right\|$	Determinante der Matrix
$\equiv \mod p$	kongruent modulo $p$ z.B. $13 \equiv 5 \mod 4$

[Bearbeiten] Geometrie

Symbol	Bedeutung
$\parallel$	parallel zu
$\perp$	senkrecht zu
$\cong$	kongruent, deckungsgleich
$\sphericalangle$	Winkel
$\left\|\sphericalangle\right\|$	Größe des Winkels

[Bearbeiten] Logik

Symbol	Bedeutung
$\hat{=}$	entspricht
$\neg$	Negation, nicht
$\wedge$	Konjunktion, und
$\vee$	Disjunktion, oder
$\Rightarrow$	Implikation (wenn ..., dann ...) z.B. $A \Rightarrow B$ (aus $A$ folgt $B$ )
$\Leftrightarrow$	Äquivalenz (genau dann , wenn ...) z.B. $A \Leftrightarrow B$ ( $A$ und $B$ sind gleichwertig)
$\overline{x}$	Logische Verneinung

[Bearbeiten] Begriffe aus der allgemeinen Mathematik

Addition

Summand + Summand = Summe

Subtraktion

Minuend - Subtrahend = Differenz

Multiplikation

$\begin{matrix} &\mathrm{Multiplikator} \cdot \mathrm{Multiplikand} &=& \mathrm{Produkt} \\ \vee &\mathrm{Faktor} \cdot \mathrm{Faktor} &=& \mathrm{Produkt} \end{matrix}$

Division

$\begin{matrix} &\mathrm{Divident} : \mathrm{Divisor} &=& \mathrm{Quotient} \\ \vee & \frac{\mathrm{Z\ddot{a}hler}} {\mathrm{Nenner}} &=& \mathrm{Quotient} \end{matrix}$

[Bearbeiten] Bruchrechnung

Addition

$\frac{a}{b}+\frac{c}{d}=\frac{ad+cb}{bd}$

Subtraktion

$\frac{a}{b}-\frac{c}{d}=\frac{ad-cb}{bd}$

Multiplikation

$\frac{a}{b}\cdot\frac{c}{d}=\frac{ac}{bd}$

Division

$\frac{a}{b}:\frac{c}{d}=\frac{ad}{bc}$

[Bearbeiten] Umrechnen von Einheiten

$\begin{matrix} 1 &\mathrm{km} =& 1000 &\mathrm{m} =& 10.000 &\mathrm{dm} =& 100.000 &\mathrm{cm} =& 1000.000 &\mathrm{mm} \\ 0,001 &\mathrm{km} =& 1 &\mathrm{m} =& 10 &\mathrm{dm} =& 100 &\mathrm{cm} =& 1000 &\mathrm{mm} \\ 0,0001 &\mathrm{km} =& 0,1 &\mathrm{m} =& 1 &\mathrm{dm} =& 10 &\mathrm{cm} =& 100 &\mathrm{mm} \\ 0,00001 &\mathrm{km} =& 0,01 &\mathrm{m} =& 0,1 &\mathrm{dm} =& 1 &\mathrm{cm} =& 10 &\mathrm{mm} \\ 0,000001 &\mathrm{km} =& 0,001 &\mathrm{m} =& 0,01 &\mathrm{dm} =& 0,1 &\mathrm{cm} =& 1 &\mathrm{mm} \end{matrix}$

Bei Flächen werden die Umrechnungen quadriert.
Bei Volumen werden die Umrechnnugen „hoch 3“ gerechnet.

[Bearbeiten] Gewichte

$\begin{matrix} 1 & \mathrm{t} =& 1000 & \mathrm{kg} & & & & & & \\ & & 1 & \mathrm{kg} &=& 1000 &\mathrm{g} &=& & \\ & & & &=& 1 &\mathrm{g} &=& 1000&\mathrm{mg} \end{matrix}$

Volumen

1dm 3 = 1l = 1000ml = 1000cm 3

[Bearbeiten] Zeit

$\begin{matrix} 1 & \mathrm{Jahr} =& 365 & \mathrm{Tage} & & & & & & \\ & & 1 & \mathrm{Tag} &=& 24 &\mathrm{Stunden} & & & \\ & & & &=& 1 &\mathrm{Stunde} &=& 60\mathrm{Minuten}& \\ & & & & & & & & 1 \mathrm{Minute} &= 60 \mathrm{Sekunden} \end{matrix}$

[Bearbeiten] Flächeninhalte

$\begin{matrix} 1 & \mathrm{km}^2 =& 100 & \mathrm{ha} & & & & & & \\ & & 1 & \mathrm{ha} &=& 100 &\mathrm{a} && & \\ & & & &=& 1 &\mathrm{a} &=& 100\mathrm{m}^2& \\ & & & & & & & & 1 \mathrm{m}^2 &= 100 \mathrm{dm}^2 \end{matrix}$

[Bearbeiten] Flächen- und Volumen-Trick

Längen quadrieren / ins Kubik nehmen z.B.

$\left(1\mathrm{m}\right)^2=\left(10\mathrm{dm}\right)^2 \Leftrightarrow 1^2\mathrm{m}^2=10^2\mathrm{dm}^2 \Leftrightarrow 1 \mathrm{m}^2=100 \mathrm{dm}^2$

[Bearbeiten] Geometrie

[Bearbeiten] Quadrat

$\begin{matrix} A&=&a^2\\ U&=&4\cdot a \end{matrix}$

[Bearbeiten] Rechteck

$\begin{matrix} A&=&a \cdot b\\ U&=&2 \cdot a+ 2 \cdot b= 2\cdot (a+b) \end{matrix}$

[Bearbeiten] Dreieck

$\begin{matrix} A&=&\frac{ch}{2}=\frac{1}{2}\cdot c \cdot h\\ U&=&a +b+c \end{matrix}$

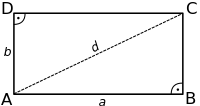
[Bearbeiten] Trapez

$\begin{matrix} A&=&\frac{a+c}{2}\cdot h\\ U&=&a +b+c+d \end{matrix}$

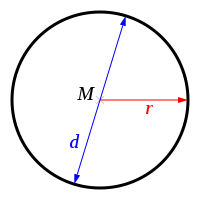
[Bearbeiten] Kreis

$\begin{matrix} A&=&\pi \cdot r^2&=&\pi \cdot \left( \frac{d}{2}\right)^2&=\pi \cdot \frac{d^2}{4}\\ U&=& 2 \pi r &=& \pi \cdot d& \end{matrix}$

[Bearbeiten] Kreissegment

$\begin{matrix} A&=&\pi r^2 \cdot \frac{\alpha}{360^\circ} \\ U&=& \pi r \cdot \frac{\alpha}{180} \end{matrix}$

[Bearbeiten] Kreisring

$\begin{matrix} A&=&\pi \left( r_2^2-r_1^2 \right)\\ U&=&2 \pi \left( r_1+r_2\right) \end{matrix}$

[Bearbeiten] Kegel

$\begin{matrix} V&=&\frac{\pi r^2 h}{3}\\ O&=&\pi r^2 + \pi \cdot r \cdot s\\ M&=&\pi \cdot r \cdot s& \end{matrix}$

[Bearbeiten] Kegelstumpf

$\begin{matrix} V&=&\frac{\pi h}{3} \cdot \left(r_1^2+r_1r_2+r_2^2\right) \\ O&=&\pi \left( r_1^2+r_2^2\right)+\pi s \cdot \left( r_1+r_2\right)\\ M&=&\pi s \cdot \left( r_1+r_2\right) \end{matrix}$

[Bearbeiten] Kugel

$\begin{matrix} V&=&\frac{4}{3} \pi r^3\\ O&=&4 \pi r^2\\ \end{matrix}$

[Bearbeiten] Zylinder

$\begin{matrix} V&=&\pi r^2 h\\ O&=&2G+M=2 \pi r \left( h+r\right)\\ M&=& 2 \pi r \cdot h= \pi d \cdot h \end{matrix}$

[Bearbeiten] Pyramide

$\begin{matrix} V&=&\frac{1}{3} a^2 h\\ O&=&2 a s + a^2\\ M&=&4\cdot \frac{as}{2}= 2 a s \end{matrix}$

[Bearbeiten] Pyramidenstumpf

$\begin{matrix} V&=&\frac{1}{3}h\left( a_1^2+a_1a_2+a_2^2\right)\\ O&=&a_1^2+a_2^2+2 s\left(a_1+a_2 \right)\\ M&=&2 s\left(a_1+a_2 \right) \end{matrix}$

[Bearbeiten] Vieleck

Ein Vieleck mit n Ecken nennt man auch n-Eck. Der Umfang eines n-Ecks berechnet sich durch:

$U = a + b + c + ... + (n - 1) + n$

Der Flächeninhalt eines n-Ecks wird durch Flächenzerlegung berechnet. Beispiel am regelmäßigen 6-Eck.

Wir betrachten, das Dreieck das aus dem Mittelpunkt des Sechsecks sowie zwei benachbarten Eckpunkten gebildet wird. Aus der Innenwinkelsumme sowie der Symmertrie der Figur ergibt sich, dass die beiden Innenwinkel des Dreiecks an den Eckpunkten des Sechsecks je $60^\circ$ betragen. Das Dreick ist daher gleichseitig. Nun zweichen wir eine Höhe in dieses Dreick ein. Womit es in zwei Dreiecke aufgeteilt ist. Wir betrachten eines dieser Dreiecke. Es ist rechtwinklig und hat. Die Hypothenusen $h$ und $\frac{a}{2}$ sowie die Kathete $a$ . Nun berechnen wir $h$ durch den Satz des Pythagoras:

$h=\sqrt{a^2-\left( \frac{a}{2}\right)^2}=\sqrt{\frac{3}{4}a^2}=\frac{1}{2}\sqrt{3}\cdot a$

Nun berechnen wir die Fläche des gleichseitigen Dreiecks durch Grundseite mal Höhe durch Zwei:

$\frac{1}{2}gh=\frac{1}{2}a \cdot \frac{1}{2}\sqrt{3}\cdot a= \frac{1}{4}\sqrt{3}a^2$

Da das Sechseck in insgesamt sechs derartige Flächen zerlegt werden kann, ergibt sich die Fläche des Sechsecks zu:

$A=6\cdot \frac{1}{4}\sqrt{3}a^2= \frac{3}{2}\sqrt{3}a^2$

[Bearbeiten] Prozentrechnung

Prozentsatz = p %

Grundwert = G

Prozentwert = W

$\begin{matrix} &W&=&G\cdot p % = G \cdot \frac{p}{100} \\ \Leftrightarrow& G&=& \frac{W \cdot 100}{p} \Leftrightarrow& p&=& \frac{W \cdot 100}{G} \end{matrix}$

[Bearbeiten] Zinsrechnung

Zinssatz = p %

Zinssatz = z %

Kapital = K

Zinswert = Z

$\begin{matrix} &Z&=&K\cdot p % = K \cdot \frac{p}{100} \\ \Leftrightarrow& K&=& \frac{Z \cdot 100}{p} \Leftrightarrow& p&=& \frac{Z \cdot 100}{K} \end{matrix}$

Tage = t

Monate = m

$\begin{matrix} &Z&=&K\cdot p % \cdot \frac{t}{360} = K\cdot p % \cdot \frac{m}{12} \\ \Leftrightarrow& K&=& \frac{Z \cdot 36000}{p\cdot t}=\frac{Z \cdot 1200}{p\cdot m} \Leftrightarrow& p&=& \frac{Z \cdot 36000}{K\cdot t}=\frac{Z \cdot 1200}{K\cdot m} \end{matrix}$

[Bearbeiten] Zinseszinz

neues Kapital = K n

Anfangskapital = K 0

Jahre = n

$\begin{matrix} &K_n&=&K_0 \cdot \left(1+ p % \right)^n= K_0 \cdot \left( 1 +\frac{p}{100}\right)^n \\ \Leftrightarrow& K_0&=& \frac{K_n }{ \left(1+ p % \right)^n }= \frac{K_n }{ \left( 1 +\frac{p}{100}\right)^n }\\ \Leftrightarrow& p&=& \left(\sqrt[n] {\frac{K_n}{K_0}}-1\right) \cdot 100 \end{matrix}$

[Bearbeiten] Lineare Funktionen

Allgemeine Funktionsgleichung:

$\begin{matrix} g(x)&=&m\cdot x +b\\ y&=&m\cdot x +b \end{matrix}$

$m$ nennt man die Steigung der linearen Funktion. $b$ nennt man Achsenabschnitt Ursprung oder Anfangswert.

$g 1$ started bei $0m$ und läuft mit $5\frac{\mathrm{m}}{\mathrm{s}}$
$g 2$ started bei $20m$ und läuft mit $7\frac{\mathrm{m}}{\mathrm{s}}$ in die andere Richtung

$\begin{matrix} g_1(x)&=& 5x \\ g_2(x)&=&-7x+20 \end{matrix}$

[Bearbeiten] Schnittpunkt

$\begin{matrix} 5x&=&-7x+20&|& +7x\\ 12x&=&20 &|& :12\\ x&=&\frac{20}{12}=\frac{5}{3}=1{,}\overline{6}\mathrm{s}&&\\ g(x)&=&\frac{25}{3}=8{,}\overline{3}\mathrm{m}&& \end{matrix}$

[Bearbeiten] Lösungverfahren

Zur Bestimmung des Schnittpunkte linearer Gleichungen. Sie können auch mit anderen Funktionen mit mehreren Variablen verwendet werden.

[Bearbeiten] Additionsverfahren

$\begin{array}{c} \left. \begin{matrix} \mathrm{I}&y&=&10x+1 &\\ \mathrm{II}&y&=&-5x+10 &| \cdot 2 \end{matrix} \right\rbrace +\\ \hline \begin{matrix} \mathrm{I}&y&=&10x+1 &\\ \mathrm{II\cdot 2}\text{ }&2y&=&-10x+20 & \end{matrix} \\ \hline \begin{matrix} \mathrm{III}&&3y&=&0x+21 &\\ &\Leftrightarrow&3y&=&21 & | :3\\ &\Leftrightarrow&y&=&7 & \end{matrix} \\ \end{array}$

einsetzen:

$\begin{matrix} y=7 &\Rightarrow &10x+1&=&7& | -1 \\ &\Leftrightarrow& 10x&=&6 & |:10\\ &\Leftrightarrow &x&= &0,1& \end{matrix}$

[Bearbeiten] Einsetzungsverfahren

$\begin{matrix} \mathrm{I}&y&=&10x+1 &\\ \mathrm{II}&x&=&-\frac{1}{5}y+2 &| \cdot 2 \end{matrix}$

$II$ einsetzten in $I$ :

$y=10\cdot \left( -\frac{1}{5}y+2 \right)+1$

ausmultiplizieren

$\begin{matrix} &y&=&-2y+20 +1 &|& +2y \\ \Leftrightarrow& 3y&=&21 &|& :3 \\ \Leftrightarrow& y&=&7 && \end{matrix}$

einsetzen:

$y=7 \Rightarrow x=-\frac{1}{5}\cdot 7+2 = -\frac{7}{5}+ \frac{10}{5}= \frac{3}{5}=0,6$

[Bearbeiten] Gleichsetzungsverfahren

$\begin{matrix} \mathrm{I}&y&=&10x+1 &\\ \mathrm{II}&y&=&-5x+10 & \end{matrix}$

Gleichsetzen $y = y$

$\begin{matrix} &10x+1&=&-5x+10 &|& +5x\\ \Leftrightarrow &15x+1&=&10 &|&-1\\ \Leftrightarrow&15x&=&9 &|&:15\\ \Leftrightarrow&x&=&0,6 \end{matrix}$

einsetzen:

$x=0.6 \Rightarrow y= 10 \cdot 0,6 +1 =6+1=7$

[Bearbeiten] Mehrere Variable

Bei mehreren Variablen braucht man soviele Gleichungen wie Variablen

$\begin{array}{c} \begin{matrix} \mathrm{I}& 2x+3y+4z&=&11 \\ \mathrm{II}&3x+4y+z&=&11\\ \mathrm{III}&4x+2y+3z&=&13\\ \end{matrix}\\ \hline \begin{matrix} \mathrm{I}& 2x+3y+4x&=&11 & \Leftrightarrow \mathrm{I} \\ 3\cdot\mathrm{I}-2\cdot\mathrm{II} &y+10z&=&11 &\Leftrightarrow \mathrm{IV}\\ -2\cdot\mathrm{I}+\mathrm{II}&-4y-5z&=&-9 & \Leftrightarrow \mathrm{V}\\ \end{matrix}\\ \hline \begin{matrix} \mathrm{I}& 2x+3y+4z&=&11 \\ \mathrm{IV} &y+10z&=&11 \\ 4\cdot\mathrm{IV}+\mathrm{V} &35z&=&35 &\Leftrightarrow z=1 \end{matrix} \end{array}$

einsetzen in $I V$ :

$\begin{matrix} &y+10&=&11 &| -10 \\ \Leftrightarrow& y&=&1 \end{matrix}$

einsetzen in $I$ :

$\begin{matrix} &2x+3+4&=&11 &|& -7 \\ \Leftrightarrow& 2x&=&4 &|& :2 \\ \Leftrightarrow& x&=&2 \\ \end{matrix}$

Probe (in $II$ oder $III$ )

$\begin{matrix} \mathrm{II}& 3\cdot 2+4\cdot 1+ 1\cdot 1&=&11 \\ &6+4+1&=&11\\ &11&=&11\\ \end{matrix}$

[Bearbeiten] Quadratische Funktionen

allgemeine Form: $f (x) = a x 2 + b x + c$

Scheitelpunktform: $a \left(x+d\right)^2+e$ mit $S \left( -d | e\right)$

der „a“ Faktor
$a < 0$	die Parabel ist nach unten geöffnet
$a > 0$	die Parabel ist nach oben geöffnet
$\| a \| = 1$	Normalparabel
$\| a \| > 1$	gestreckte (schmale) Parabel
$\| a \| < 1$	gestauchte (breite) Parabel

Umwandlung aus der algemeinen Form in die Scheitelpunktform:

$\begin{matrix} f(x)&=&ax^2+bx+c &|& \text{a ausklammern} \\ f(x)&=&a \left(x^2+\frac{b}{a}x+\frac{c}{a}\right) &|& \text{quadratische }\mathrm{Erg\ddot anzung} \\ f(x)&=&a \left(x^2+\frac{b}{a}x+ \underbrace{\left( \frac{b}{2a}\right)^2-\left( \frac{b}{2a}\right)^2}_{\text{quadratische }\mathrm{Erg\ddot anzung}}+\frac{c}{a}\right) && \end{matrix}$

Das nennt man quadratische Ergänzung, da man den „ $b$ “ Wert aus der binomischen Formel „dazuergänzt“. Um den Term nicht zu veränderen wird diese Ergänzung sofort wieder subtrahiert.

Es gibt die erste Binomische Formel:

$x^2+\frac{b}{a}x+\left(\frac{b}{2a}\right)^2=\left( x+\frac{b}{2a}\right)^2$

$\begin{matrix} &\Rightarrow &f(x)&=&a\left( \left( x+\frac{b}{2a}\right)^2- \left( \frac{b}{2a}\right)^2 +\frac{c}{a} \right) &| \mathrm{ausmultiplizieren} \\ &\Leftrightarrow& f(x)&=&a\left( x+\underbrace{\frac{b}{2a}}_{d}\right)^2- \left( \underbrace{\frac{b^2}{4a}-c}_{c}\right)& \end{matrix}$

Aus der Form $f(x)=a\left( \left( x+\frac{b}{2a}\right)^2- \left( \frac{b}{2a}\right)^2 +\frac{c}{a} \right)$ kommen wir leicht zu Nullstellenberechnung: $f (x) = 0$

$\begin{matrix} &&0&=&a\left( \left( x+\frac{b}{2a}\right)^2- \left( \frac{b}{2a}\right)^2 +\frac{c}{a} \right) &|& :a\\ &\Leftrightarrow &0&=& \left( x+\frac{b}{2a}\right)^2- \left( \frac{b}{2a}\right)^2 +\frac{c}{a} &|&-\frac{c}{a}+\left(\frac{b}{2a}\right)^2 \\ &\Leftrightarrow& \left(\frac{b}{2a}\right)^2-\frac{c}{a} &=& \left( x+\frac{b}{2a}\right)^{\overset{\text{ }}{2}} &|& \sqrt{\text{ }} \\ &\Leftrightarrow&\pm \sqrt{\left(\frac{b}{2a}\right)^2-\frac{c}{a}}&=&x+\frac{b}{2a} &|& - \frac{b}{2a} \\ &\Leftrightarrow& x_{1,2}&=&- \frac{b}{2a} \pm \sqrt{\left(\frac{b}{2a}\right)^2-\frac{c}{a}} & & \end{matrix}$

Die Formel ist auch unter dem Namen $p q$ -Formel bekannt, wegen $\frac{b}{a}=p \wedge \frac{c}{a}=q$

$\Rightarrow x_{1,2}=-\frac{p}{2}\pm \sqrt{(\left( \frac{p}{2}\right)^2-q)}$

Einige Parabelbeispiele

Auch hier gibt es wieder einiges zu berechnen:

Nullpunkt (die Punkte an denen die Parabel die $x$ -Achse schneidet.) $\rightarrow pq$ -Formel.
Scheitelpunkte (die $x$ und $y$ Werte an die Parabel ihr Minimum bzw. Maximum annimmt) $\rightarrow$ quadratische Ergänzung
Schnittpunke zweier Parabeln. $\rightarrow$ Genau wie beim Schnitt zweier Geraden geht man auch hier davon aus, dass beide Parabeln an ihren Schnittpunkten gleiche $x$ und $y$ Werte haben müssen. Daher sagen wir auch hier: $\begin{matrix} &&f(x)_1=f(x)_2 \\ &\Leftrightarrow& a_1 x^2 + b_1 x + c_1= a_2 x^2 + b_2 x + c_3 \end{matrix}$

[Bearbeiten] Natürliche Zahlen

Natürliche Zahlen sind die Zahlen, die wie der Name schon sagt in der Natur vorkommen. Das heißt, sie sind „anfaßbar“.

z.B. 1 Apfel, 2 Personen, 10,- €

Die Menge der natürlichen Zahlen wird mit $\mathbb{N}$ gekennzeichnet. $\in \mathbb{N}$ (gesprochen: Element von N).

$\mathbb{N}^*$ ist die Menge der natürlichen Zahlen inklusive $0$ (auch $\mathbb{N}_0$ ).

[Bearbeiten] Schriftliche Addition

Bei großen Zahlen empfielt es sich ofmals die Addition schriftlich durchzuführen. Dazu schreibt man die zu berechnenden Zahlen übereinander (beginnend von rechts), so dass die Einer über den Einern stehen und die Zehner über den Zehneren usw. Diese rechnet man von recht beginnend, von oben nach unten zusammen. Unten notiert man nur die Einer der errechneten Zahl. Den Rest addiert man zur nächsten Zahl usw.

z.B.

$\begin{array}{c|c|c|c|c|c|c} &1&3&9&4&8&6\\ +& & & &9&8&3\\ +& & &4&2&1&0\\ +& & & & & &2\\ +& &8&7&9&6&4\\ \hline =&\overset{1}{2}&\overset{2}{3}&\overset{2}{2}&\overset{2}{6}&\overset{1}{4}&5\\ \hline \hline \end{array}$

Nebenrechnung:

$\begin{matrix} 6+3+2+4&=&15 &\text{ }(\text{5 ist der Einer}) \\ 8+8+1+6+1&=&24 &\\ 4+9+2+9+2&=&26 & \\ 9+4+7+2&=&22 &\\ 3+8+2&=&13& \\ 1+1&=&2& \end{matrix}$

[Bearbeiten] Schriftliche Subtraktion

Die Schriftlich Subtraktrion funktioniert ähnlich der Addition. Auch hier schreiben wir die zu subtrahierenden Zahlen untereinander. Dann addiren wir alle übereinander stehenden Ziffern um diese dann von der entsprechenden Ziffer des Minuenden zu subtrahieren. Falls diese Summe größer ist als die entsprechende Ziffer des Minuenden, so wird ihr ein Zehner hinzugefügt, dieser wird jedoch zugleich als einer von der Ziffer links daneben subtrahiert. z.B. $\begin{array}{cc|c|c|c|c|c|c|c|c} &9&8&7&6&5&4&3&2&1\\ \hline -& & & &9&4&3&8&0&0 \\ -& & & & &2&1&7&6&8 \\ -& & & & & & & &9&1 \\ -& & &\underset{1}{\text{ }} &\underset{1}{\text{ }}&\underset{1}{\text{ }}&\underset{2}{\text{ }}&\underset{2}{5}&\underset{1}{1}&0 \\ \hline &9&8&6&6&8&8&1&5&2 \\ \hline \hline \end{array}$

Nebenrechnung:

$\begin{matrix} &0+8+1+0&=&9 \\ &1-9&=&\text{geht nicht}\\ \text{daher}&11-9 &=&2 \\ &0+6+9+1+1=17 \\ &2-17 &=&\text{geht nicht}\\ \text{daher}&12-17 &=&\text{geht nicht} \\ \text{daher}&22-17 &=&5 \\ &8+7+5+2=22 \\ &3-22 &=&\text{geht nicht}\\ \text{daher}&13-22 &=&\text{geht nicht} \\ \text{daher}&23-22 &=&1 \\ &3+1+2=6 \\ &4-6 &=&\text{geht nicht}\\ \text{daher}&14-6 &=&8 \\ &4+2+1=7 \\ &5-7 &=&\text{geht nicht}\\ \text{daher}&15-7 &=&8 \\ &9+1=10 \\ &6-10 &=&\text{geht nicht}\\ \text{daher}&16-10 &=&6 \\ &1&=&1\\ &7-1 &=&6\\ \end{matrix}$

Beispiel 2:

$\begin{array}{cc|c|c|c} &4&3&2&1\\ \hline +& &2&1&0\\ -& & &9&1\\ -& &\underset{1}{1}&\underset{1}{0}&1\\ \hline &4&3&3&9\\ \hline \hline \end{array}$

Nebenrechnung:

$\begin{matrix} 1+0&=&1 &\oplus \\ 1+1&=&2 &\ominus \\ 1-2&=&\text{geht nicht} \\ 11-2&=&9 \\ 2+1&=&3 &\oplus \\ 9+0+1&=&10 &\ominus \\ 3-10&=&\text{geht nicht} \\ 13-10&=&3 \\ 11-2&=&9 \\ 3+2&=&5 &\oplus \\ 1+1&=&2 &\ominus \\ 5-2&=&3 \\ 4-0&=&4 \end{matrix}$

[Bearbeiten] Schriftliche Multiplikation

Auch die schriftliche Multiplikation funktioniert nach dem Prinzip der schriftlichen Addition. Bloß wir hier nicht addiert sonden multipliziert.

$\begin{array}{c|c|c|c|c|c|c|c|c|c} 1&2&3&4&5&6&\cdot&3&7&8 \\ \hline & & & &\overset{1}{9}&\overset{2}{8}&\overset{3}{7}&\overset{4}{6}&\overset{4}{4}&8\\ & & & \overset{1}{8}&\overset{2}{6}&\overset{3}{4}&\overset{3}{1}&\overset{4}{9}&2&\\ & &3&\overset{1}{7}&\overset{1}{0}&\overset{1}{3}&\overset{1}{6}&8&&\\ \hline & &\overset{1}{4}&\overset{1}{6}&\overset{1}{6}&\overset{1}{6}&\overset{2}{6}&3&6&8 \\ \hline \hline \end{array}$

Nebenrechung:

Man beginnt mit den beiden Ziffern ganz rechts. Also mit 6 und 8.

$6 \cdot 8= 48$

Die 8 kommt in das Kästchen ganz rechts oben. Links daneben kommt die 4. Jedoch nicht in die mitte des Kästchens sondern als kleine Ziffer an den oberen Rand des Kästchens.

Nun geht man in der ersten Zahl von der 6 Ziffer links von ihr. Also zur 5.

$5 \cdot 8= 40$

Zu diesem Ergebniss addiert man die Ziffer die wir gerade an den oberen Rand des Kästchens geschrieben haben. Also 4.

$40 + 4 = 44$

Die 4 auf der Einerstelle kommt nun in die mitte der Kästenchens links von der 8. Die 4 auf der Zehnerstelle kommt Links daneben an den oberen Rand des Kästchens. Danch wir dieses Verfahren immer weiter wiederholt.

$\begin{matrix} 8\cdot4 &=&32 &&32+4&=&\overline{3}6 \\ 8\cdot 3 &=& 24 && 24+\overline{3}&=&\underline{2}7 \\ 8\cdot 2&=& 16 &&16+\underline{2}&=&18\\ 8\cdot 1 &=& 8 &&8+1&=&9 \end{matrix}$

Durch die Unter- bzw. Überstriche wurde angedeutet, dass die Ziffer 3 aus der ersten Zeile in der zweiten Zeile der Zahl 24 hinzuaddiert wird, bzw. die Ziffer 2 aus der zweiten Zeile der 16 in der dritten Zeile der Ziffer 16 hinzuaddiert wird. Analoges gilt natürlich auch für die Ziffer 1 der Zahl 18 in der dritten Zeile.

In den folgenden Zeilen verfährt man analog. Schließlich addiert man, die Ergebnisse auf, wie im Abschnitt über schriftliche addition beschrieben.

[Bearbeiten] Schriftliche Division

Es kommt schon mal vor, dass wir mit Zahlen rechnen, die nicht mehr Elemente der Natürlichen Zahlen sind. Die passiert schnell wenn man Zahlen teilen muss. Hierzu teilen wir nacheinander (von links nach rechts) eine Zahl durch einen Divisor. Geht diese nicht mehr, so setzen wir ein Komma im Divisor und ziehen der zu teilenden Zahl eine 0 hinzu.

$\begin{array}{c|c|c|c|c|c|ccc} 9&8&4&3&:&1&2&=&820{,}25 \\ 9&6& & & & & & & \\ \hline &2&4& & & & & & \\ &2&4& & & & & & \\ \hline & &0&3& & & & & \\ & &0&0& & & & & \\ \hline & &0&3&0& & & & \\ & & &2&4& & & & \\ \hline & & & &6&0& & & \\ & & & &6&0& & & \\ \hline & & & & &0& & & \\ \end{array}$

Benutzer:Dirk Huenniger/Mathe

Aus Wikibooks

Inhaltsverzeichnis

[Bearbeiten] Das griechische Alphabet

[Bearbeiten] Mathematische Symbole

[Bearbeiten] Mengenlehre

[Bearbeiten] Algebra

[Bearbeiten] Geometrie

[Bearbeiten] Logik

[Bearbeiten] Begriffe aus der allgemeinen Mathematik

[Bearbeiten] Bruchrechnung

[Bearbeiten] Umrechnen von Einheiten

[Bearbeiten] Längen

[Bearbeiten] Gewichte

[Bearbeiten] Zeit

[Bearbeiten] Flächeninhalte

[Bearbeiten] Flächen- und Volumen-Trick

[Bearbeiten] Geometrie

[Bearbeiten] Quadrat

[Bearbeiten] Rechteck

[Bearbeiten] Dreieck

[Bearbeiten] Trapez

[Bearbeiten] Kreis

[Bearbeiten] Kreissegment

[Bearbeiten] Kreisring

[Bearbeiten] Kegel

[Bearbeiten] Kegelstumpf

[Bearbeiten] Kugel

[Bearbeiten] Zylinder

[Bearbeiten] Pyramide

[Bearbeiten] Pyramidenstumpf

[Bearbeiten] Vieleck

[Bearbeiten] Prozentrechnung

[Bearbeiten] Zinsrechnung

[Bearbeiten] Zinseszinz

[Bearbeiten] Lineare Funktionen

[Bearbeiten] Schnittpunkt

[Bearbeiten] Lösungverfahren

[Bearbeiten] Additionsverfahren

[Bearbeiten] Einsetzungsverfahren

[Bearbeiten] Gleichsetzungsverfahren

[Bearbeiten] Mehrere Variable

[Bearbeiten] Quadratische Funktionen

[Bearbeiten] Natürliche Zahlen

[Bearbeiten] Schriftliche Addition

[Bearbeiten] Schriftliche Subtraktion

[Bearbeiten] Schriftliche Multiplikation

[Bearbeiten] Schriftliche Division

Ansichten

Persönliche Werkzeuge

Navigation

Suche

Mitmachen

Werkzeuge

Drucken/exportieren