Differenz, symmetrische Differenz und Komplement – Serlo „Mathe für Nicht-Freaks“

Differenz

Erklärungen zur Differenzmenge (Youtube-Video der KhanAcademyDeutsch)

Mit Hilfe der Differenz kannst du alle Elemente einer Menge $A$ bestimmen, die außerdem nicht in einer zweiten Menge $B$ liegen. Ein Objekt $x$ liegt genau dann in der Differenz von $A$ und $B$, wenn $x$ ein Element von $A$ aber kein Element von $B$ ist:

Differenz zweier Mengen

Für die Differenz von $A$ und $B$ schreibt man $A\setminus B$ (ausgesprochen: „$A$ ohne $B$“). Seltener wird in der Literatur ein Minuszeichen verwendet: $A-B$.

Definition (Differenz)

Die Differenz $A\setminus B$ zweier Mengen $A$ und $B$ ist definiert durch:

$A\setminus B:=\{x\,|\,x\in A\land x\notin B\}$

Beispiel (Differenz)

• $\{2,4,5,9\}\setminus \{1,3,5,7,12\}=\{2,4,9\}$
• $\{3,12,18\}\setminus \{1,5,7\}=\{3,12,18\}$
• $\{x\in \mathbb {N} |\,x{\text{ gerade}}\}\setminus \{x\in \mathbb {N} |\,x{\text{ ungerade}}\}=\{x\in \mathbb {N} |\,x{\text{ gerade}}\}$

Symmetrische Differenz

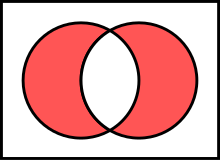
symmetrische Differenz zweier Mengen

Beispiel für die symmetrische Differenz von zwei Mengen

Die Symmetrische Differenz zweier Mengen $A$ und $B$ ist die Menge aller Objekte, die in genau einer der Mengen $A$ und $B$ enthalten sind. Ist also $x$ ein Element aus der symmetrischen Differenz von $A$ und $B$, so ist entweder $x\in A$ oder $x\in B$ und $x$ kein gemeinsames Element der Mengen $A$ und $B$. Ihre Schreibweise ist $A\,\triangle \,B$ (ausgesprochen: „symmetrische Differenz von $A$ und $B$“).

Definition (symmetrische Differenz)

Die symmetrische Differenz $A\,\triangle \,B$ ist die Menge aller Objekte, die Elemente genau einer der Mengen $A$ und $B$ sind:

${\begin{aligned}A\,\triangle \,B&:=(A\setminus B)\cup (B\setminus A)\\[0.3em]&=\{x\in A\cup B|\,(x\in A\land x\notin B)\lor (x\notin A\land x\in B)\}\\[0.3em]&=\{x\in A\cup B|x\in A\,{\dot {\lor }}\,x\in B\}\\[0.3em]\end{aligned}}$

Verständnisfrage: Bilde die symmetrische Differenz folgender Mengen

1. $\{1,2,3\}\,\triangle \,\{2,3,4\}=?$
2. $\{1,2,3\}\,\triangle \,\{1,2,3\}=?$
3. $\{1,2,3\}\,\triangle \,\{4,5,6\}=?$
4. $\mathbb {Z} \,\triangle \,\mathbb {R} =?$
5. $\mathbb {N} \,\triangle \,\varnothing =?$

Antwort:

1. $\{1,2,3\}\,\triangle \,\{2,3,4\}=\{1,4\}$
2. $\{1,2,3\}\,\triangle \,\{1,2,3\}=\emptyset$
3. $\{1,2,3\}\,\triangle \,\{4,5,6\}=\{1,2,3,4,5,6\}$
4. $\mathbb {Z} \,\triangle \,\mathbb {R} =\mathbb {R} \setminus \mathbb {Z} =\left\{x\in \mathbb {R} \,|\,x{\text{ ist keine ganze Zahl.}}\right\}$
5. $\mathbb {N} \,\triangle \,\varnothing =\mathbb {N}$

Komplement

das Komplement einer Menge

Erklärungen zur Grundmenge und dem absoluten Komplement (Youtube-Video der KhanAcademyDeutsch)

Das Komplement einer Menge $A$ ist die Menge aller Objekte der Grundmenge, die keine Elemente von $A$ sind. Ist in einem Text keine Grundmenge angegeben, so ergibt sich diese aus dem Kontext. So wird bei einem Text über die reelle Analysis die Grundmenge meistens die Menge der reellen Zahlen $\mathbb {R}$ sein. Da wir hier über Mengen reden, verwenden wir die Allklasse ${\mathcal {V}}$ anstelle der Grundmenge. Die Schreibweise für das Komplement von $A$ ist $A^{\complement }$ oder $\complement A$ oder ${\overline {A}}$ (ausgesprochen: „Komplement von $A$“).

Definition (Komplement)

Das Komplement $A^{\complement }$ der Menge $A$ ist die Menge aller Objekte, die nicht in $A$ enthalten sind:

$A^{\complement }:=\{x\,|\,x\notin A\}$

Verständnisfrage: Die Grundmenge sei $\mathbb {N} _{0}$. Bilde folgende Komplemente:

1. $\{0,1,2,3\}^{\complement }=?$
2. $\left\{x\in \mathbb {N} _{0}\,|\,x{\text{ ist gerade}}\right\}^{\complement }=?$
3. $\mathbb {N} _{0}^{\complement }=?$
4. $\varnothing ^{\complement }=?$

Antwort:

1. $\{0,1,2,3\}^{\complement }=\mathbb {N} _{\geq 4}$
2. $\left\{x\in \mathbb {N} _{0}\,|\,x{\text{ ist gerade}}\right\}^{\complement }=\left\{x\in \mathbb {N} _{0}\,|\,x{\text{ ist ungerade}}\right\}$
3. $\mathbb {N} _{0}^{\complement }=\varnothing$
4. $\varnothing ^{\complement }=\mathbb {N} _{0}$

Satz (Eigenschaften des Komplements)

1. $(A^{\complement })^{\complement }=A$ (Doppeltes Komplement)
2. $A\cap A^{\complement }=\varnothing$
3. $A\cup A^{\complement }={\mathcal {V}}$
4. $(A\cap B)^{\complement }=A^{\complement }\cup B^{\complement }$ (De Morgansche Regel)
5. $(A\cup B)^{\complement }=A^{\complement }\cap B^{\complement }$
6. ${\mathcal {V}}^{\complement }=\varnothing$
7. $\varnothing ^{\complement }={\mathcal {V}}$

Dieses Eigenschaften lassen sich auf die Definitionen und die entsprechenden Gesetze der Logik zurückführen.

Beweis (Eigenschaften des Komplements)

1. Es ist
   ${\begin{aligned}&(A^{\complement })^{\complement }\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Definition\;von\;\complement }}\right.}\\[0.3em]=\;&\{y\,|\,y\notin A\}^{\complement }\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Definition\;von\;\complement }}\right.}\\[0.3em]=\;&\{x\,|\,x\notin \{y\,|\,y\notin A\}\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Abstraktionsprinzip\;f{\ddot {u}}r\;x\notin \{y\,|\,y\notin A\}}}\right.}\\[0.3em]=\;&\{x\,|\,x\in A\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Extensionalit{\ddot {a}}tsprinzip}}\right.}\\[0.3em]=\;&A\end{aligned}}$
2. Es ist
   ${\begin{aligned}&A\cap A^{\complement }\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Definition\;von\;\complement }}\right.}\\[0.3em]=\;&A\cap \{y|\,y\notin A\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Definition\;von\;\cap }}\right.}\\[0.3em]=\;&\{x\;|\;x\in A\land x\in \{y|\,y\notin A\}\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Abstraktionsprinzip\;f{\ddot {u}}r\;x\notin \{y\,|\,y\notin A\}}}\right.}\\[0.3em]=\;&\{x\;|\;x\in A\land x\notin A\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {die\;Konjunktion\;ist\;immer\;falsch}}\right.}\\[0.3em]=\;&\{x\;|\;x\neq x\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Definition\;von\;\varnothing }}\right.}\\[0.3em]=\;&\varnothing \end{aligned}}$
3. Siehe Aufgabe 1
4. Es ist
   ${\begin{aligned}&(A\cap B)^{\complement }\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Definition\;von\;\complement }}\right.}\\[0.3em]=\;&\{x\;|\;x\notin A\cap B\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Definition\;von\;\cap }}\right.}\\[0.3em]=\;&\{x\;|\;x\notin \{y\;|\;y\in A\land y\in B\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Abstraktionsprinzip\;f{\ddot {u}}r\;x\notin \{y\,|\,y\in A\land y\in B\}}}\right.}\\[0.3em]=\;&\{x\;|\;\neg \,(x\in A\land x\in B)\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {de\;Morgansche\;Regel\;f{\ddot {u}}r\;\neg \;und\;\land }}\right.}\\[0.3em]=\;&\{x\;|\;x\notin A\lor x\notin B\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Abstraktionsprinzip\;f{\ddot {u}}r\;x\notin A\;und\;x\notin B}}\right.}\\[0.3em]=\;&\{x\;|\;x\in \{y\,|y\notin A\}\lor x\in \{z\,|\,z\notin B\}\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Definition\;von\;\complement }}\right.}\\[0.3em]=\;&\{x\;|\;x\in A^{\complement }\lor x\in B^{\complement }\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Definition\;von\;\cap }}\right.}\\[0.3em]=\;&A^{\complement }\cup B^{\complement }\end{aligned}}$
5. Siehe Aufgabe 2
6. Es ist
   ${\begin{aligned}&{\mathcal {V}}^{\complement }\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Definition\;von\;\complement }}\right.}\\[0.3em]=\;&\{x\,|\,x\notin {\mathcal {V}}\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ x\notin {\mathcal {V}}\;{\mathsf {ist\;immer\;falsch}}\right.}\\[0.3em]=\;&\{x\,|\,x\neq x\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Definition\;von\;\varnothing }}\right.}\\[0.3em]=\;&\varnothing \end{aligned}}$
7. Siehe Aufgabe 3

Übungsaufgaben

Aufgabe (Aufgabe 1)

Zeige: $A\cup A^{\complement }={\mathcal {V}}$

Lösung (Aufgabe 1)

${\begin{aligned}&A\cup A^{\complement }\\[0.3em]&{\color {NavyBlue}\left\updownarrow \ {\mathsf {Definition\;von\;\complement }}\right.}\\[0.3em]=\;&A\cup \{y|\,y\notin A\}\\[0.3em]&{\color {NavyBlue}\left\updownarrow \ {\mathsf {Definition\;von\;\cup }}\right.}\\[0.3em]=\;&\{x\;|\;x\in A\lor x\in \{y|\,y\notin A\}\}\\[0.3em]&{\color {NavyBlue}\left\updownarrow \ {\mathsf {Abstraktionsprinzip\;f{\ddot {u}}r\;x\notin \{y\,|\,y\notin A\}}}\right.}\\[0.3em]=\;&\{x\;|\;x\in A\lor x\notin A\}\\[0.3em]&{\color {NavyBlue}\left\updownarrow \ {\mathsf {die\;Disjunktion\;ist\;immer\;wahr}}\right.}\\[0.3em]=\;&\{x\;|\;x=x\}\\[0.3em]&{\color {NavyBlue}\left\updownarrow \ {\mathsf {Definition\;von\;{\mathcal {V}}}}\right.}\\[0.3em]=\;&{\mathcal {V}}\end{aligned}}$

Aufgabe (Aufgabe 2)

Zeige: $(A\cup B)^{\complement }=A^{\complement }\cap B^{\complement }$

Lösung (Aufgabe 2)

${\begin{aligned}&(A\cup B)^{\complement }\\[0.3em]&{\color {NavyBlue}\left\updownarrow \ {\mathsf {Definition\;von\;\complement }}\right.}\\[0.3em]=\;&\{x\;|\;x\notin A\cup B\}\\[0.3em]&{\color {NavyBlue}\left\updownarrow \ {\mathsf {Definition\;von\;\cup }}\right.}\\[0.3em]=\;&\{x\;|\;x\notin \{y\;|\;y\in A\lor y\in B\}\\[0.3em]&{\color {NavyBlue}\left\updownarrow \ {\mathsf {Abstraktionsprinzip\;f{\ddot {u}}r\;x\notin \{y\,|\,y\in A\lor y\in B\}}}\right.}\\[0.3em]=\;&\{x\;|\;\neg \,(x\in A\lor x\in B)\}\\[0.3em]&{\color {NavyBlue}\left\updownarrow \ {\mathsf {de\;Morgansche\;Regel\;f{\ddot {u}}r\;\neg \;und\;\lor }}\right.}\\[0.3em]=\;&\{x\;|\;x\notin A\land x\notin B\}\\[0.3em]&{\color {NavyBlue}\left\updownarrow \ {\mathsf {Abstraktionsprinzip\;f{\ddot {u}}r\;x\notin A\;und\;x\notin B}}\right.}\\[0.3em]=\;&\{x\;|\;x\in \{y\,|y\notin A\}\land x\in \{z\,|\,z\notin B\}\}\\[0.3em]&{\color {NavyBlue}\left\updownarrow \ {\mathsf {Definition\;von\;\complement }}\right.}\\[0.3em]=\;&\{x\;|\;x\in A^{\complement }\land x\in B^{\complement }\}\\[0.3em]&{\color {NavyBlue}\left\updownarrow \ {\mathsf {Definition\;von\;\cap }}\right.}\\[0.3em]=\;&A^{\complement }\cap B^{\complement }\end{aligned}}$

Aufgabe 3: Zeige: $\varnothing ^{\complement }={\mathcal {V}}$

${\begin{aligned}&\varnothing ^{\complement }\\[0.3em]&{\color {NavyBlue}\left\updownarrow \ {\mathsf {Definition\;von\;\complement }}\right.}\\[0.3em]=\;&\{x\,|\,x\notin \varnothing \}\\[0.3em]&{\color {NavyBlue}\left\updownarrow \ x\notin \varnothing \;{\mathsf {ist\;immer\;wahr}}\right.}\\[0.3em]=\;&\{x\,|\,x=x\}\\[0.3em]&{\color {NavyBlue}\left\updownarrow \ {\mathsf {Definition\;von\;{\mathcal {V}}}}\right.}\\[0.3em]=\;&{\mathcal {V}}\end{aligned}}$