Existence of a measure continuation – Serlo
In this chapter we deal with the question when a continuation of a function on sets to a measure (which is a special function on sets) exists and consider how such a continuation can be constructed. We learn about -subadditivity and exterior measures. We will derive the theorem of Carathéodory and the measure continuation theorem.
First thoughts
[Bearbeiten]Under what conditions can a set function defined on any set system be continued to a measure on the generated -algebra ? We will find the answer to this question step by step in this chapter.
A simple example will soon show that the whole thing is a bit more complicated as it first seems. Shouldn't it be intuitively sufficient that a function defined on a set system has some nice measure properties to (somehow) continue it to a measure? (Meaning, a measure on the generated -algebra ?) The set function has the measure properties if and -additivity hold. In other words, if is a pre-measure on . That this condition is necessary is clear: if is not a pre-measure on the set system , then in particular no continuation of can be a pre-measure and hence also not a measure.
So must be a pre-measure on . But this is unfortunately not sufficient: may have properties that preclude making it a volume or a measure, for instance because monotonicity is violated. We saw an example of this at the very beginning in the article volumes on rings. However, the property of -additivity can be trivially satisfied simply because there are no disjoint sets in .
Example (A -additive, but not monotonic function on sets)
with
Therefore, let's first go back a few steps and recall our very first considerations about measurement functions.
Sub-additive function on sets
[Bearbeiten]In the article volumes on rings, we introduced volume-measuring functions as extensive quantities. So doubling the size of the underlying system doubles the quantity (as for volume, mass, energy, etc.). As a central common feature of all extensive volume-measuring functions we have observed the monotonicity, i.e. the property that an increase of the size of a set / an object / a quantity also leads to an increase of the measurement result. We have generalized the monotonicity to the subadditivity, from which one can infer the monotonicity. The subadditivity says that a superset of some within a set system has more volume than the set itself. This makes sense for extensive quantities. We recall the definition:
Definition (Sub-additivity of a function on sets)
A function on sets is called (finitely) sub-additive (or subadditive), if for all and with it holds that:
Only later we supplemented this extensive property by the "exact" property of additivity and in this context described the set ring as a possible domain of definition of volume-measuring functions. On set rings it was sufficient to require only the additivity to infer also subadditivity and monotonicity. But in general these two properties of a function on sets do not follow from additivity if the domain of definition has no further structure, as the example above has shown. Therefore, our first goal will be to construct a subadditive continuation of defined on a set system, which is as large as possible.
Construction of an outer volume
[Bearbeiten]A subadditive set function can be interpreted as an outer approximation: If a set of sets is covered, then its volume is less than or equal to the volume of the covering sets. It does not matter whether the sets are disjoint with respect to the set or whether they cover only "roughly":
-
A set is split into sets .
-
The sets cover , but are not disjoint.
In some cases, the sum of the volumes of a disjoint aprtition might be larger than the union. This is indeed allowed for an outer approximation. We admit "upward deviations" for the the volume. This makes subadditivity easier to control under continuations, compared to additivity, and it is even possible to have a subadditive continuation on all of . On the other hand, an additive (instead of just subadditive) set function cannot generally be defined on the entire power set , as we saw in the Example of Banach-Tarski. This means that not every subset of the basic set is "exactly" measurable. However, every arbitrary set can be outer-approximated.
So let us use this connection between subadditivity and the approximation of sets by covers in the construction of a subadditive continuation. Let be a subadditive function defined on the set system . Of course, must also hold. Therefore, we can assume without restriction by defining (even if should hold). Using approximating covers, we now construct a subadditive continuation defined on the power set: for a set covered by sets (i.e., ), we define
Problem: The value of depends on the selected cover!
Example
Consider the set system and the function on it . For the set , both and are supersets (=outer approximations) from . The value is then correspondingly or . Intuitively, the second variant is better, as it reproduces the measure exactly and the first is just an approximation.
So for to be well-defined, we must choose among all possible supersets from . We choose for every set the value for that belongs to that superset (=covering) of , which approximates the best. Because we approximate from above (outer approximation), this is just the covering that gives the smallest value for . There may be infinitely many possible coverings, so a minimum might not exist and we generalize to taking the infimum:
Hint
The infimum of the empty set is defined by (the empty set has no largest lower bound). This corresponds to the case where is too "large" and it cannot be covered by sets from .
Possibly there is no covering that gives exactly the value (only values that are slightly too large will come out), but by definition of the infimum one can approximate the value arbitrarily close.
Following the construction with the infimum we have for every and every arbitrary covering with sets that:
In the estimate above, we used that the are contained in the set of covers of which is used to for the infimum.
This inequality is already very similar to our goal: we want to obtain subadditivity for the outer approximations. However,we still have an on the left-hand side and a uon the right-hand side. It remains to establish a similar inequality with on both sides, which will be out subadditivity for .
Theorem
Let be a set system with and a function on it with . Then the function defined on the power set:
is then subadditive and further we have .
Proof
We first prove that .
Since is a covering of the empty set with sets from , we know that the -value is smaller than the -value . But -values are always positive, i.e., for all . So which means .
Now we turn to subadditivity. Let be arbitrary with . We have to show that holds.
Let without restriction for all , because otherwise the inequality will hold anyway. The idea now is to trace the covering of back to a covering with sets from . For this we can exploit that it is contained in the set of coverings over which the infimum is formed, and thus obtain an upper bound. For this we choose for every single a covering with sets from (which exists because ). Then
is a covering of . After construction of we thus have
The second inequality involves summing over the sets from , as in the sum before, with the difference that some sets may be counted twice. (Here we used that is non-negative!)
Now we want to obtain something of the form
To do this, we need to reduce the coverings of the to the while sustaining the inequality. In other words, we need
However, the construction of only guarantees
But since we can arbitrarily approximate the value of the infimum in the defining equation of , we can choose the coverings to be only slightly larger than . So let . To every we choose such that:
Then we obtain from the above estimate
and since was arbitrary, we get
Hint
Note that in the theorem we did not require the subadditivity of . For to be subadditive, need not itself be subadditive. To show the theorem, it suffices that is a nonnegative function on sets with . We will nevertheless need the subadditivity of in a moment, namely to prove that is indeed a continuation of .
We now have a subadditive function defined on all of , namely with . Following the considerations on outer approximation, we call a set function defined on the power set with these properties an outer volume.
Definition (Outer volume)
A function is called an outer volume, if:
- ,
- is subadditive.
Warning
This term is not used in the literature.
Note also: What we call here "outer volume" is not a volume in the proper sense, because it is in general not additive.
There still remains the question whether is continued by , i.e. whether for all . So far, we know , because one of many coverings of . But we don't know yet if there is no "lower" approximation and that the infimum over all possible covers does not yield a smaller value. Intuitively, this should be true, but we have to prove it. So what we want is: for all . This is the case if for all finite coverings of with sets we have that . In other words, we additionally need the subadditivity of on .
Theorem
Let be a set system with , let be a subadditive function on it with and let be defined as above. Then is a continuation of .
Proof
We have to show that for all . Since is covered by we have by definition of as being the infimum over all coverings. Conversely we have that for any with also , which follows from the subadditivity of . Since this is satisfied for all coverings of , we have that the inequality also holds for the infimum over all possible coverings and finally, we obtain .
To make clear that the outer volume defined as above is a continuation of , we write for it. In summary, we have proved:
Theorem (about constructing an outer volume)
Let be a set system with and a subadditive function on it with . Then
is an outer volume and a continuation of .
Warning
In general, the set function defined in the theorem is one possible continuation of to an outer volume. There may be many others. We will deal with the question of the uniqueness of a continuation later on.
Finally, let us consider a short example. The outer Jordan volume is defined starting from the geometric volume in :
Example (Outer Jordan-volume)
A (half-open) cuboid aggregates is a finite union of half-open cuboids of the form .

We consider the set system
of the cuboid aggregates in . This set system is a ring. On the ring of cuboid aggregates we consider the geometric volume , which assigns to a cuboid its volume
Every cuboid aggregate can also be written as a disjoint union of finitely many cuboids, and its volume is the sum of the individual cuboid volumes. (Note that it is always possible to write as a disjoint union of half-open cuboids, and that the value does not depend on the choice of the decomposition). The set function defined in this way is called Jordan volume. One can show that on the set system is indeed additive, that is, a volume. In particular we have that and since is a ring, the additivity of also implies subadditivity.
We can construct the outer Jordan volume by defining for any subset:
According to what we just proved, is indeed an outer volume (subadditive) and a continuation of . This approximates the volume of arbitrary subsets of by covering them with cuboid aggregates.

From outer volumes to volumes
[Bearbeiten]We have identified subadditivity as an essential property in the previous section and put it in the focus of the construction of a continuation. But do our considerations about subadditivity (constructing outer volumes) lead us to the final goal (constructing volumes) and if so, how can we reach it? We are still interested in an "exact" (additive) or additionally continuous (-additive) extension of the measure - on a domain which shall be as large as possible. A subadditive continuation to the whole power set (what we have) is of course fine, but we are interested an additive or -additive continuation, which might only be possible on a smaller set system than .
Let's go step by step and take care of the additivity first. If we can find out the sets on which a subadditive set function behaves additively, we have already taken a good step: By simply restricting the domain of definition to those sets, we already get a volume. And from that point, it is not far to a measure (hopefully only taking a limit , but let's see).
So the goal now is to restrict the domain of definition and thus make additive on it - so it is a volume. Now, how do we find out on which sets, is additive?
Carathéodory measurability condition
[Bearbeiten]We are looking for those where the is "exact", i.e.:
These are two unknown variables ( and ) in one expression, so ti is difficult to handle. We keep only as the unknown quantity and consider (and thus also ) as arbitrarily given and known. We cannot simplify anything on the left side of the equation. So let's try to express on the right side all expressions with by and :
Since we know nothing about , we must allow for all sets with . Thus, for the sets on which is additive, we would like:
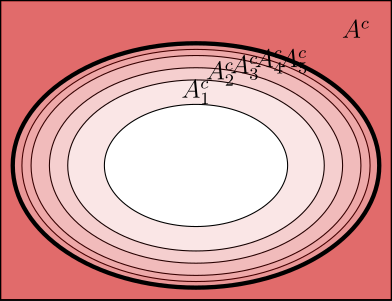
Let's recall the considerations about the approximation with coverings and the additivity as a property to have an "exact" statement of the volume. Then intuitively the sets for which the condition is fulfilled can be approximated. Approximability of a set can be interpreted as the property that approximation of outside and of inside coincide, just as for integrable functions the values for upper and lower approximation should converge to the same limit. If we understand the inner approximation of a set as an outer approximation of the complement (see picture), it follows from this consideration that if a set is measurable, then also its complement should be measurable.
-
Inner approximation of the set by .
-
Outer approximation of the set by .
This equivalence is already expressed in the above formula, because due to it is symmetric in and :
But now we have required that the equation be satisfied only for those with . If a set is measurable, then because of we cannot yet infer the measurability of the complement . To establish the desired symmetry between the approximability of a set and its complement , the defining equation of measurability must be satisfied for any . The sets for which the equation holds for all , are the sets "exactly" approximated by , therefore these are also called -measurable sets. This condition of measurability was introduced by the mathematician Constantin Carathéodory and is named after him.
Definition (Messurability condition of C. Carathéodory)
Let be an outer volume. A set is called -measurable, if for all it holds that:
The set is called set system of all -measurable sets.
Hint
Often the equation in the measurability condition is formulated equivalently as:
Sometimes you may find "" instead of "", i.e.,
By subadditivity, we have that "" holds anyway.
Warning
In the literature, the measurability condition is defined for so-called outer measures instead of outer volumes. We will get to know outer measures later, and our definition of measurability carries over to them without changes.
Application of the measurability criterion
[Bearbeiten]We invented a condition which is intended to allow us to find the sets on which an outer volume is additive. And indeed this works. The proof is not difficult:
Theorem
Let be an outer volume. Then is additive on .
Proof
We prove the statement for two disjoint sets. Then, additivity for arbitrarily finitely many pairwise disjoint sets follows by induction.
Let be disjoint.Because of the measurability of , we have:
Just as well, of course, one can argue with the measurability of .
We can even already say something about the structure of :
Theorem
Let be an outer volume. Then, we have:
Proof
The first two properties follow almost immediately from the measurability definition:
Let be arbitrary. Because of and we have that:
Let now . Using the equivalent measurability condition , we see that for symmetry reasons .
Finally, let and be arbitrary. Multiple application of the property "-measurable subset" yields:
So we already have a -algebra without "": all that is missing is the closedness with respect to countably infinite unions. A set system with these properties is accordingly called an algebra:

Definition (Algebra)
A set system is called an algebra, if:
Inductively, from follows naturally the closedness with respect to any finite union.
Remember: every algebra is a ring. Every ring with is an algebra.
In summary, we found that:
Theorem (Intermediate result)
Let be an outer content. Then is an algebra and is finitely additive, so it is a volume on it.
-
Recall: A -algebra contains the basic set and all limiting sets within it.
-
Recall: An algebra (of sets) also must contain . But limits of sets need not be included.
-sub-additivity and outer volumes
[Bearbeiten]At this point, we have come quite far. Only the -additivity and closure with respect to countable unions is missing in order to get a measure. It is tempting to just take the limit and conclude that we have the same properties for countably infinite sets. But it is not clear whether subadditivity (as generalized monotonicity) is preserved when going over to limit values!
Example (Outer Jordan-volume)
Let be the outer Jordan volume on , which we already met in a more general form for in an example above. The set system of cuboid aggregates in considered there corresponds here to the set of all finite unions of half-open intervals of the form . From the above example we know that is indeed an outer volume, that is, finitely subadditive. Furthermore, if we consider sets of the form as approximating coverings for one-point sets , we see that
It follows that assigns the geometric length not only to half-open but also to arbitrary intervals (because the boundary points have length 0). We now consider the set .
Since is subadditive (cf. the Example above), we have that for every finite covering of with sets :
However, this inequality is no longer true, when taking countably infinite coverings.
It is easy to see that every finite covering of with sets must cover all of since is dense in . So the most exact possible covering of is obviously the interval itself, and thus we have that . Let now be an enumeration of the elements of (this set is countable). Then . However,
Thus, the subadditivity is not preserved in the transition to infinite covers!
Historical note: To consider countably infinite covers instead of finite ones in the definition of the outer Jordan volume was the decisive idea in the development of the integration theory by Lebesgue. We will see shortly why.
So the reasoning we used in the previous section for finite additivity may not work if we want additivity for infinitely many disjoint sets. This is because in order for us to infer -additivity and stability under countable unions, it is necessary that subadditivity is also preserved under limit values. In other words, the inequality in the definition of subadditivity should also hold for coverings with (countably) infinite sets. The example shows that this is not true in general and has to be required separately. So we extend the notion of subadditivity to coverings with countably many sets and call this new property -subadditivity:
Definition (-sub-additivity of a function on sets)
A function is called -subadditive, if for all with it holds that:
Before we investigate whether we reach our goal for a -additive set function on , we introduce a new name for outer volumes with this property. We called subadditive set functions defined on the power set with outer volumes earlier. The turns a "volume" into a "measure". So it is natural to call a -subadditive set function with an outer measure.
Definition (Outer measure)
A function is called an outer measure, if it holds that
- ,
- is -subadditive.
Warning
Again an outer measure is not a measure in the proper sense, for it is generally not -additive on .
Because , every outer measure is also finitely subadditive (and hence monotonic). We can just choose as covering sets.
Construction of an outer measure
[Bearbeiten]Let us recall the extension "without the " we constructed above. Maybe, it is possible to copy some useful ideas from it.
We started from a function defined on any set system with and . Then we constructed an outer volume , which we did using finite coverings that approximate by sets from :
The set function defined this way is actually subadditive on the entire power set with , so it is an outer volume. We have seen that it is a continuation of , meaning that it coincides with on the set system , provided that also has the properties of an outer volume, i.e. it is subadditive on in addition to .
Now we want to construct a -subadditive set function defined on the power set with . We call a set function with these properties an outer measure. Thus, it should also hold for countably infinite covers of a set , , that
When proving the subadditivity of the outer volume constructed above, we exploited that the value is given by the infimum over all possible finite coverings of a set . From the definition we immediately obtained an inequality very similar to subadditivity
for a set covered by sets . However, we cannot expect from the above that it is also -subadditive: The infimum is formed only over finite coverings, and it is not guaranteed that the inequality is preserved when moving to countably infinite coverings. With the Jordan volume on and the set we have already seen an example for this.
So to be able to infer the -subadditivity of and to get the inequality also for infinite covers, we must form the infimum also over these and correspondingly tweak the definition, such that it becomes:
Note that since we have also included finite covers: choose . The set function thus constructed is in fact -subadditive, and the proof is analogous to the proof of subadditivity of the outer volume constructed above:
Theorem
Let be a set system with and a function on it with . Then the functio defined on the power set:
is an outer measure.
Proof
We establish the two properties of an outer measure. First that and then the subadditivity. For this we note the following: let be a covering of , i.e. let . Then, we have because of
that, by definition of ,
Since, moreover, because of the nonnegativity of for any all elements in are nonnegative, we have .
By considering the covering of , it follows with these two statements directly, that
Now we turn to -subadditivity. Let for this be any set sequence . We will show that .
W.l.o.g, let for all . Now if for any , then because it follows also that . So we get
Let now be arbitrary.
From the definition of using the infimum it follows for every that there is a covering of with sets , such that
Since every single one of the sequences covers the set .
That is, is a countable covering of consisting of sets in . From this follows
Since this inequality holds for all , a limit transition implies the assertion
For the same reasons as for the construction of an outer volume, must have the properties of an outer measure on to be continued by :
Theorem
Let be a set system with , let be a -subadditive function on it with and let be defined as above. Then, is a continuation of .
Proof
Let . We now prove . Since is covered by , we have .
We show the other inequality. Let be any cover of . Then,from the -subadditivity of we obtain
Since this holds for all coverings there is also
To denote that the outer measure defined above is a continuation of , we write for it. In summary, we have proved:
Theorem (Constructing an outer measure)
Let be a set system with and a -subadditive function on it with . Then,
is an outer measure continuing .
Warning
Note that the function constructed in the theorem is one continuation of to an outer measure. It does not need to be the only one.
From an outer measure to a measure
[Bearbeiten]Equipped with the additional property of -additivity, we can now also show that is a -algebra and is even -additive on it. The proof is based on taking a limit starting from the intermediate result to finite additivity and going to countably infinite additivity:
Theorem
Let be an outer measure. Then is a -algebra.
Proof
Since every outer measure is in particular an outer volume, the intermediate result above holds and we know that is an algebra. So we only have to prove the closure of with respect to countably infinite unions, i.e. that unions of measurable sets are measurable again. So what we want for all and all is:
The main work is to prove "", because "" follows already from finite subadditivity. We first show the statement for infinite unions of pairwise disjoint sets from . For this, let be pairwise disjoint. Then, for all :
In the first two equations we used that finite unions of sets from lie again in and that behaves finitely additively on . The last estimate comes from the monotonicity of , as
Since the inequality above holds for all , it is preserved in the limit :
Note that it was really necessary to exploit finite additivity before taking the limit, to make it clear that the inequality is preserved! Now is an outer measure, so it is -subadditive, and we get from a further estimate of the first summand
where the last inequality follows from finite subadditivity. So we have equality everywhere. Hence, we proved measurability of for pairwise disjoint .

To obtain the statement for arbitrary countably infinite unions, we again use the intermediate result already proved, according to which is an algebra. To make a union "artificially" disjoint, we take, one after another, each set and "cut out" the part which is already contained in the union of the first sets .
For this we use the relation . Now, an algebra is intersection-, union- and complement-stable, so:
Then is a union of pairwise disjoint sets from and lies in , as well. So for non-disjoint it alos holds that
which concludes the proof.
From the above estimate, by appropriate choice of , the -additivity of on directly follows:
Theorem
Let be an outer measure. Then is -additive on .
Proof
Let be pairwise disjoint. In the proof above, using -subadditivity for disjoint sets, we obtained the following estimate:
so equality holds everywhere and
for all . The choice of establishes the claim.
So, in summary, we have proved the following theorem, named after the mathematician Constantin Carathéodory:
Theorem (by C. Carathéodory, 1914)
Let be an outer measure. Then is a -algebra and a measure on it.
Intermediate conclusion of results
[Bearbeiten]Let us conclude the above definitions and results:
Construction of an outer measure
[Bearbeiten]We have learned the property of -subadditivity. It generalizes subadditivity to coverings (approximations) of a set with countably infinite sets and can be understood as a form of subadditivity which is preserved when passing to limits.
Definition (-aub-additivity of a function on sets)
A function is called -subadditive, if for all with it holds that:
An outer measure is a -subadditive function defined on the entire power set. It can be interpreted as an approximation of the volume of the subsets of , but is in general not a measure (not -additive) on the power set.
Definition (Outer measure)
A function is called an outer measure, if:
- ,
- is -subadditive.
One can construct an outer measure out of nearly every set function defined on some set system. The idea of construction is to understand the property of an outer measure as an outer approximation by coverings that should be as precise as possible. If itself has the properties of an outer measure (-subadditivity), then the outer measure constructed out of it is a continuation of .
Theorem (Construction of an outer measure)
Let be a set system with and a function on it with . Then the set function defined on the power set
is an outer measure. If is additionally -subadditive on , then this outer measure is a continuation of and we also write for it.
Theorem of Carathéodory
[Bearbeiten]Using the measurability condition of Carathéodory one can find those sets on which an external measure is even additive. The sets for which the condition is fulfilled can be understood as sets which can be exactly approximated (or measured) by the outer measure.
Definition (Measurability condition of C. Carathéodory)
Let be an outer measure. A set is called -measurable, if for all it holds that:
The set is called the set of -measurable sets.
The set of measurable sets with respect to an outer measure is a -algebra. Further, an outer measure on the set of measurable sets is a measure: By definition of the measurability condition, a subadditive set function on the measurable sets behaves additively. If the subadditivity is preserved even in the transition to the limit, then from -subadditivity before taking the limit, we can infer -additivity after taking the limit.
Theorem (C. Carathéodory, 1914)
Let Be an outer measure. Then is a -algebra and a measure on it.
The continuation theorem
[Bearbeiten]We now know: If is a -subadditive function defined on a set system with , then we can use it to construct an outer measure on the power set which continues . For this we define for any :
We can define the sets measurable with respect to an outer measure. These form a -algebra and the outer measure is -additive (a measure) to it. In what follows we keep the names , and and use them in the same sense.
The goal now is to apply these results to construct the continuation. For this we want to restrict to the -algebra generated by and finally get a measure on . What still has to be done is to prove that the original set system is really contained in the -algebra of the -measurable sets. So each original set in is indeed -measurable.
If this was not the case, we would be in serious trouble: Just restricting the to -measurable sets would then not work, since , implies . So the -algebra would be "too small", then.
Conversely, if , then , wecause the -opearator jast picks the smallest -algebra larger than and is such a -algebra.
So what we need to continue to a measure on is the measurability of sets in . In other words,
should hold for all and all . So we need a further condition on .
However, instead of simply requiring the above equation as a condition, we will use a slightly weaker condition of measurability of the sets from , which is still sufficient. For this we approximate an arbitrary set by sets . Then it suffices for the measurability of a set to require that the above equality holds only for all sets , rather than for any .
So let be any set, for which we want to show measurabilit. Let further be arbitrary and let such that
The aim is to show
by exploiting that the equation is satisfied for the in place of . From subadditivity and -subadditivity of the external measure , we conclude:
Now we have assumed that the measurability equation is satisfied for sets in . This holds for all as the are from :
The last equality holds, since the outer measure was constructed as a continuation of (see above) and on it agrees with . It follows that
Now note that by definition of we can approximate the value by appropriate choice of covers of to arbitrary precision. Let thus and let be chosen such that
It follows together with the estimates made above that
and taking the limit we get measurability of .
Thus we have finally shown this result for the measurability of the sets from :
Theorem
Let be a function which is continued by an outer measure ,
If for every
holds for any , then the sets from are -measurable.
Finding a suitable condition for the measurability of the sets from was the last step to construct a continuation of to a measure on the -algebra generated by the set system . This finally allows us to write down the main theorem about measure continuation:
Theorem (Continuation to a measure)
Let be a set system with and a -subadditive function defined on it with . Let further
the canonical continuation of to an outer measure. If for every is the equation
is satisfied for any , then , restricted to the generated algebra , defines a measure that continues .
Alternative versions of the continuation theorem
[Bearbeiten]Often, the literature states the measure continuation theorem in other versions than the one formulated by us. Often not of an arbitrary set system (with ) is assumed, but additionally a certain structure is presupposed. This is especially useful with respect to the uniqueness of a continuation, because only for sufficiently "large" set systems , the measure which continues a set function is uniquely determined by the values on . This question will concern us in detail in the next chapter "Uniqueness of a continuation". Here we will only briefly consider alternative formulations of the continuation theorem.
If the set system is stable under taking differences, then the condition formulated by us in the continuation theorem for the measurability of the sets from is equivalent to (and hence also ) being additive on . This is the content of the following little theorem.
Theorem
Let denote a set system stable under taking differences (e.g. a ring). As before, let denote a function defined on and denote the continuation of to a measure. Then the following are equivalent:
- is additive on .
Proof
First "": let be additive on and let be arbitrary. Since is additive on with , also is additive on and we have that:
Now to "". This direction follows directly from the measurability condition as characterization of the additivity. Let be disjoint and set . Then, we have by assumption:
Thus, if we assume that is a ring, we can replace the somewhat unwieldy condition for measurability of sets from with additivity in our version of the continuation theorem. (Instead of a ring, one can of course substitute any set system stable under differences (e.g. an algebra)). In fact, even a "restricted" stability under differences, as in the case of semi-rings is sufficient. Semi-rings are set systems which have somewhat less structure than rings. In particular, the difference of two sets does not necessarily lie in the semi-ring again, but can always be written as a disjoint union of finitely many sets from the set system.
Example (Semi-ring)
The set of half-open rectangles is a semi-ring on .

From this semi-ring, the ring of cuboid aggregates is generated, which we already encountered in an example above. This ring contains all figures aggregates of finitely many half-open rectangles.

One can show that the continuation of a (-)additive set function of a semi-ring on the ring generated by it is always possible while preserving the (-)additivity. This justifies the following variant of the continuation theorem:
Theorem (Continuation theorem, alternative version)
Let be a semi-ring and an additive, -subadditive set function with . Then there exists a continuation of to a measure on .
(A German reference, where this version can be found, is Achim Klenke: Wahrscheinlichkeitstheorie. 2., korrigierte Auflage.)
We can also show that a volume on a ring is -subadditive if and only if it is -additive (a premeasure). So if is a ring (or an algebra, because every algebra is a ring), then we can summarize additivity and -subadditivity in our version of the continuation theorem as follows:
Theorem (Continuation theorem, alternative version)
Let be a ring/algebra and a premeasure (definition right above the theorem). Then there exists a continuation of to a measure on .
However, the assumptions made here are relatively strong. Set rings can be large (for example, the ring of cuboid aggregates), so it may be difficult to prescribe all values of a premeasure on it. Here the following, also often used variant of the continuation theorem helps, which gets along with somewhat weaker assumptions. Also here the reason lies in the fact that a continuation of a semi-ring on the ring generated by it is possible under preservation of the -additivity.
Theorem (Continuation theorem, alternative Version)
Let be a semi-ring and a premeasure. Then there exists a continuation of to a measure on .
(A German reference, where this version can be found, is Jürgen Elstrodt: Maß- and Integrationstheorie. 8. Auflage.)





![{\displaystyle \mu :{\mathcal {C}}\to [0,\infty ]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/8e6ea20cff9642fed34b67c6f1e7f1d976a3751b)




![{\displaystyle \mu :\{\{1\},\{1,2\}\}\to [0,\infty ]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/c7ebf576dfa81d11deb0babd48f10c49bd902b44)


![{\displaystyle \mu :{\mathcal {P}}(\Omega )\supseteq {\mathcal {C}}\to [0,\infty ]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/768b75e26239b05258496d94d0cb1457b4e61bee)


















![{\displaystyle {\mathcal {C}}=\{(a,b]:a,b\in \mathbb {R} \}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/b699de5823deb28ed04d65a3bfaf41de7fce764b)
![{\displaystyle \mu :{\mathcal {C}}\rightarrow [0,\infty ],\;\mu ((a,b]):=b-a}](https://wikimedia.org/api/rest_v1/media/math/render/svg/4a0302a27189a9f744c35f1850c8da8c7a7258e4)
![{\displaystyle A:=(1,2]\cup (3,4]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/e8998e013ebf9a47f60add6327cb5d2c49afba0c)
![{\displaystyle (-5,5]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/a85bbeb44a077963f75ba6acc1190ea0c7560752)
![{\displaystyle (1,2]\cup (3,4]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/8f753e88ecc01780c64675aac436a5f0af24f4b4)









![{\displaystyle \eta :{\mathcal {P}}(\Omega )\to [0,\infty ],\quad \eta (A):=\inf \left\{\sum _{i=1}^{n}\mu (C_{i}):n\in \mathbb {N} ,C_{1},\dots ,C_{n}\in {\mathcal {C}},A\subseteq \bigcup _{i=1}^{n}C_{i}\right\}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/69d734c56c771cd3362f6c6d6c121ffdcdc061d1)





















![{\displaystyle \eta :{\mathcal {P}}(\Omega )\to [0,\infty ]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/9553e436fcc68ab56743649a94b8406f3a54ee4e)











![{\displaystyle \mu ^{*}:{\mathcal {P}}(\Omega )\to [0,\infty ],\quad \mu ^{*}(A):=\inf \left\{\sum _{i=1}^{n}\mu (C_{i}):n\in \mathbb {N} ,C_{1},\dots ,C_{n}\in {\mathcal {C}},A\subseteq \bigcup _{i=1}^{n}C_{i}\right\}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/54f93c9d123330e9c5edb76b033ceacda96905fe)


![{\displaystyle (a_{1},b_{1}]\times \dots \times (a_{n},b_{n}]\subseteq \mathbb {R} ^{n}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/d0ad18c1e81c428d41cedef833b1df2e8907e595)
![{\displaystyle {\mathcal {C}}=\left\{\bigcup _{i=1}^{m}Q_{i}\subseteq \mathbb {R} ^{n}:m\in \mathbb {N} ,Q_{j}=(a_{1}^{(j)},b_{1}^{(j)}]\times \dots \times (a_{n}^{(j)},b_{n}^{(j)}],j=1,\dots ,m\right\}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/a9cdc68866b1a1200a9385110448697c4242c257)
![{\displaystyle Q=(a_{1},b_{1}]\times \dots \times (a_{n},b_{n}]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/575a74f73bf504e28bb51e8834ab635a453c5c3e)


![{\displaystyle \mu ^{*}:{\mathcal {P}}(\mathbb {R} ^{n})\to [0,\infty ]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/9c44d724128034e4eff350fda2b52a87a1fc11c1)



































![{\displaystyle {\begin{aligned}\eta (Q)&=\eta (Q\cap A)+\eta (Q\cap A^{c})\\[0.3em]&=\eta (Q\cap A)+\eta (Q\cap A^{c}\cap B)+\eta (Q\cap A^{c}\cap B^{c})\\[0.3em]&=\eta (Q\cap (A\cup B)\cap A)+\eta (Q\cap (A\cup B)\cap A^{c})+\eta (Q\cap (A\cup B)^{c})\\[0.3em]&=\eta (Q\cap (A\cup B))+\eta (Q\cap (A\cup B)^{c})\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/36a8c8dfce45cbd832c954e3e1b69cdfb1c166e3)






![{\displaystyle (a,b]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/6a6969e731af335df071e247ee7fb331cd1a57ae)
![{\displaystyle A_{n}:=(x-1/n,x]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/99fe36aae28ef6f70a589b4f397dba9b19b1b806)


![{\displaystyle \mathbb {Q} \cap [0,1]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/822d49c61001a9fd0fee4578855b367df40dc4ca)

![{\displaystyle \eta (\mathbb {Q} \cap [0,1])\leq \sum _{i=1}^{n}\eta (A_{i})}](https://wikimedia.org/api/rest_v1/media/math/render/svg/cd0c480fe354caf35812d610954ef40c2f0ba94b)
![{\displaystyle [0,1]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/738f7d23bb2d9642bab520020873cccbef49768d)

![{\displaystyle \eta (\mathbb {Q} \cap [0,1])=1}](https://wikimedia.org/api/rest_v1/media/math/render/svg/e8f2d95378768250afe292ca7d3074af0c35aa8c)

![{\displaystyle \mathbb {Q} \cap [0,1]\subseteq \bigcup _{i=1}^{\infty }\{q_{i}\}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/c02a25ed9ff813da2f6e72df926af7fa9657c2b1)
![{\displaystyle \eta (\mathbb {Q} \cap [0,1])=1>0=\sum _{i=1}^{\infty }\eta (\{q_{i}\})}](https://wikimedia.org/api/rest_v1/media/math/render/svg/df8123928086b52dc6c04173f49fca110e39bcd6)






![{\displaystyle A=\mathbb {Q} \cap [0,1]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/ac7947dc4ed1485e3ce4e7f7aeb7ccaf8bfbf177)


![{\displaystyle \eta :{\mathcal {P}}(\Omega )\to [0,\infty ],\quad \eta (A):=\inf \left\{\sum _{i=1}^{\infty }\mu (C_{i}):C_{1},C_{2},\dots \in {\mathcal {C}},A\subseteq \bigcup _{i=1}^{\infty }C_{i}\right\}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/9f060e9942700e9957a90506ca804804adb83bb8)






















![{\displaystyle {\begin{aligned}\eta (A)&\leq \sum _{n,k\in \mathbb {N} }\mu (E_{n,k})=\sum _{n=1}^{\infty }\sum _{k=1}^{\infty }\mu (E_{n,k})\leq \sum _{n=1}^{\infty }(\eta (A_{n})+\varepsilon \cdot 2^{-n})\\[0,3em]&=\sum _{n=1}^{\infty }\eta (A_{n})+\varepsilon \cdot \sum _{n=1}^{\infty }2^{-n}=\sum _{n=1}^{\infty }\eta (A_{n})+\varepsilon .\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/4ec120e36a13ab840683712431a2da92d654e16c)









![{\displaystyle \mu ^{*}:{\mathcal {P}}(\Omega )\to [0,\infty ],\quad \eta (A):=\inf \left\{\sum _{i=1}^{\infty }\mu (C_{i}):C_{1},C_{2},\dots \in {\mathcal {C}},A\subseteq \bigcup _{i=1}^{\infty }C_{i}\right\}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/2cbed10a1201aae423ae1a5458ff173ffcf83b20)















![{\displaystyle \eta :{\mathcal {P}}(\Omega )\rightarrow [0,\infty ]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/5872a2128e61a8ad1d199b3a3768f0b366e4b849)



















![{\displaystyle \mu ^{*}:{\mathcal {P}}(\Omega )\to [0,\infty ]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/95eee293dedc2d1070c2a87cafaa081894588150)
![{\displaystyle \mu :{\mathcal {C}}\rightarrow [0,\infty ]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/06d2b7a145e4a8689a51cd6eee53f5a81a2a7946)
![{\displaystyle \mu ^{*}:{\mathcal {P}}(\Omega )\to [0,\infty ],\quad \mu ^{*}(A)=\inf \left\{\sum _{i=1}^{\infty }\mu (A_{i}):A_{1},A_{2},\dots \in {\mathcal {C}},A\subseteq \bigcup _{i=1}^{\infty }A_{i}\right\}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/18f98dc0de685da6ded4d89342182dbcb44a3034)









![{\displaystyle (a_{1},b_{1}]\times \dots \times (a_{n},b_{n}]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/8dc35460308400d1222a29ff2a5a146967866bba)
