Deine Meinung zählt – Gestalte unsere Lerninhalte mit!
Wir entwickeln neue, interaktive Formate für die Hochschulmathematik. Nimm dir maximal 15 Minuten Zeit, um an unserer Umfrage
Mit deinem Feedback machen wir die Mathematik für dich und andere Studierende leichter zugänglich!
Dieser Abschnitt ist noch im Entstehen und noch nicht offizieller Bestandteil des Buchs. Gib den Autoren Zeit, den Inhalt anzupassen!
Dieser Artikel enthält die grundlegenden und abgeleiteten Definitionen der Operationen auf Mengen sowie verschiedene Übungen zu diesen Operationen und ihren Eigenschaften.
Die Grundlegende Operationen von Mengen und die logischen Operationen stehen in engem Zusammenhang und werden in der Mathematik oft gemeinsam verwendet. Die grundlegenden Operationen von Mengen, Vereinigung, Durchschnitt und Komplement, entsprechen den grundlegenden Operationen in der mathematischen Logik: OR, AND und NOT.
→ Hauptartikel: Durchschnitt von Mengen
→ Hauptartikel: Vereinigung von Mengen
Definition (disjunkte Vereinigung)
Vereinigung zweier Polygonmengen Die Schreibweise
C
=
A
∪
˙
B
{\displaystyle C=A\,{\dot {\cup }}\,B}
(
C
=
A
∪
B
)
∧
(
A
∩
B
=
∅
)
{\displaystyle {\big (}C=A\,{\cup }\,B{\big )}\land (A\cap B=\varnothing )}
∪
˙
{\displaystyle {\dot {\cup }}}
Definition (Große Vereinigung)
Die Vereinigung über der Menge
M
{\displaystyle M}
M
{\displaystyle M}
⋃
M
:=
{
x
|
∃
y
∈
M
:
x
∈
y
}
{\displaystyle \bigcup M:=\{x\,|\,\exists y\in M:x\in y\}}
Für weitere Details können Sie den folgenden Artikel einsehen:
Differenz von Mengen
Für weitere Details können Sie den folgenden Artikel einsehen:
Komplement einer Menge
Für weitere Details können Sie den folgenden Artikel einsehen:
Lösung (Eigenschaften der Operationen von Mengen)
Lösung Teilaufgabe 1:
A
∪
A
∁
↕
D
e
f
i
n
i
t
i
o
n
v
o
n
∁
=
A
∪
{
y
|
y
∉
A
}
↕
D
e
f
i
n
i
t
i
o
n
v
o
n
∪
=
{
x
|
x
∈
A
∨
x
∈
{
y
|
y
∉
A
}
}
↕
A
b
s
t
r
a
k
t
i
o
n
s
p
r
i
n
z
i
p
f
u
¨
r
x
∉
{
y
|
y
∉
A
}
=
{
x
|
x
∈
A
∨
x
∉
A
}
↕
d
i
e
D
i
s
j
u
n
k
t
i
o
n
i
s
t
i
m
m
e
r
w
a
h
r
=
{
x
|
x
=
x
}
↕
D
e
f
i
n
i
t
i
o
n
v
o
n
V
=
V
{\displaystyle {\begin{aligned}&A\cup A^{\complement }\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Definition\;von\;^{\complement }}}\right.}\\[0.3em]=\;&A\cup \{y|\,y\notin A\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Definition\;von\;\cup }}\right.}\\[0.3em]=\;&\{x\;|\;x\in A\lor x\in \{y|\,y\notin A\}\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Abstraktionsprinzip\;f{\ddot {u}}r\;x\notin \{y\,|\,y\notin A\}}}\right.}\\[0.3em]=\;&\{x\;|\;x\in A\lor x\notin A\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {die\;Disjunktion\;ist\;immer\;wahr}}\right.}\\[0.3em]=\;&\{x\;|\;x=x\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Definition\;von\;{\mathcal {V}}}}\right.}\\[0.3em]=\;&{\mathcal {V}}\end{aligned}}}
Lösung Teilaufgabe 2:
(
A
∪
B
)
∁
↕
D
e
f
i
n
i
t
i
o
n
v
o
n
∁
=
{
x
|
x
∉
A
∪
B
}
↕
D
e
f
i
n
i
t
i
o
n
v
o
n
∪
=
{
x
|
x
∉
{
y
|
y
∈
A
∨
y
∈
B
}
↕
A
b
s
t
r
a
k
t
i
o
n
s
p
r
i
n
z
i
p
f
u
¨
r
x
∉
{
y
|
y
∈
A
∨
y
∈
B
}
=
{
x
|
¬
(
x
∈
A
∨
x
∈
B
)
}
↕
d
e
M
o
r
g
a
n
s
c
h
e
R
e
g
e
l
f
u
¨
r
¬
u
n
d
∨
=
{
x
|
x
∉
A
∧
x
∉
B
}
↕
A
b
s
t
r
a
k
t
i
o
n
s
p
r
i
n
z
i
p
f
u
¨
r
x
∉
A
u
n
d
x
∉
B
=
{
x
|
x
∈
{
y
|
y
∉
A
}
∧
x
∈
{
z
|
z
∉
B
}
}
↕
D
e
f
i
n
i
t
i
o
n
v
o
n
∁
=
{
x
|
x
∈
A
∁
∧
x
∈
B
∁
}
↕
D
e
f
i
n
i
t
i
o
n
v
o
n
∩
=
A
∁
∩
B
∁
{\displaystyle {\begin{aligned}&(A\cup B)^{\complement }\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Definition\;von\;^{\complement }}}\right.}\\[0.3em]=\;&\{x\;|\;x\notin A\cup B\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Definition\;von\;\cup }}\right.}\\[0.3em]=\;&\{x\;|\;x\notin \{y\;|\;y\in A\lor y\in B\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Abstraktionsprinzip\;f{\ddot {u}}r\;x\notin \{y\,|\,y\in A\lor y\in B\}}}\right.}\\[0.3em]=\;&\{x\;|\;\neg \,(x\in A\lor x\in B)\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {de\;Morgansche\;Regel\;f{\ddot {u}}r\;\neg \;und\;\lor }}\right.}\\[0.3em]=\;&\{x\;|\;x\notin A\land x\notin B\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Abstraktionsprinzip\;f{\ddot {u}}r\;x\notin A\;und\;x\notin B}}\right.}\\[0.3em]=\;&\{x\;|\;x\in \{y\,|y\notin A\}\land x\in \{z\,|\,z\notin B\}\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Definition\;von\;\complement }}\right.}\\[0.3em]=\;&\{x\;|\;x\in A^{\complement }\land x\in B^{\complement }\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Definition\;von\;\cap }}\right.}\\[0.3em]=\;&A^{\complement }\cap B^{\complement }\end{aligned}}}
Lösung Teilaufgabe 3:
∅
∁
↕
D
e
f
i
n
i
t
i
o
n
v
o
n
∁
=
{
x
|
x
∉
∅
}
↕
x
∉
∅
i
s
t
i
m
m
e
r
w
a
h
r
=
{
x
|
x
=
x
}
↕
D
e
f
i
n
i
t
i
o
n
v
o
n
V
=
V
{\displaystyle {\begin{aligned}&\varnothing ^{\complement }\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Definition\;von\;^{\complement }}}\right.}\\[0.3em]=\;&\{x\,|\,x\notin \varnothing \}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ x\notin \varnothing \;{\mathsf {ist\;immer\;wahr}}\right.}\\[0.3em]=\;&\{x\,|\,x=x\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Definition\;von\;{\mathcal {V}}}}\right.}\\[0.3em]=\;&{\mathcal {V}}\end{aligned}}}
Lösung Teilaufgabe 4:
(
A
∩
B
)
∪
(
A
∩
B
∁
)
↕
D
e
f
i
n
i
t
i
o
n
v
o
n
∪
=
{
x
|
x
∈
(
A
∩
B
)
∨
x
∈
(
A
∩
B
∁
)
}
↕
D
e
f
i
n
i
t
i
o
n
v
o
n
∩
=
{
x
|
(
(
x
∈
A
)
∧
(
x
∈
B
)
)
∨
(
(
x
∈
A
)
∧
(
x
∈
B
∁
)
)
}
↕
D
i
s
t
r
i
b
u
t
i
v
g
e
s
e
t
z
v
o
n
∧
a
n
∨
=
{
x
|
(
x
∈
A
)
∧
(
(
x
∈
B
)
∨
(
x
∈
B
∁
)
)
}
↕
E
i
g
e
n
s
c
h
a
f
t
v
o
n
∨
=
{
x
|
(
x
∈
A
)
∧
T
}
↕
E
i
g
e
n
s
c
h
a
f
t
v
o
n
∧
=
{
x
|
(
x
∈
A
)
}
=
A
{\displaystyle {\begin{aligned}&(A\cap B)\cup (A\cap B^{\complement })\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Definition\;von\;\cup }}\right.}\\[0.3em]=\;&\{x\,|\,x\in (A\cap B)\,\lor \,x\in (A\cap B^{\complement })\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Definition\;von\;\cap }}\right.}\\[0.3em]=\;&\{x\,|\,((x\in A)\land (x\in B))\,\lor \,((x\in A)\land (x\in B^{\complement }))\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Distributivgesetz\;von\;\land \;an\;\lor }}\right.}\\[0.3em]=\;&\{x\,|\,(x\in A)\land ((x\in B)\,\lor \,(x\in B^{\complement }))\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Eigenschaft\;von\;\lor }}\right.}\\[0.3em]=\;&\{x\,|\,(x\in A)\land T\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Eigenschaft\;von\;\land }}\right.}\\[0.3em]=\;&\{x\,|\,(x\in A)\}\\[0.3em]=\;&A\end{aligned}}}
Lösung Teilaufgabe 5:
A
∩
(
B
∖
A
)
↕
D
e
f
i
n
i
t
i
o
n
v
o
n
∩
=
{
x
|
x
∈
A
∧
(
x
∈
B
∖
A
)
}
↕
D
e
f
i
n
i
t
i
o
n
v
o
n
∖
=
{
x
|
x
∈
A
∧
x
∈
B
∧
x
∉
A
}
↕
d
i
e
K
o
n
j
u
n
k
t
i
o
n
i
s
t
i
m
m
e
r
f
a
l
s
c
h
=
{
x
|
F
∧
x
∈
B
}
↕
E
i
g
e
n
s
c
h
a
f
t
v
o
n
∧
=
∅
{\displaystyle {\begin{aligned}&A\cap (B\setminus A)\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Definition\;von\;\cap }}\right.}\\[0.3em]=\;&\{x\,|\,x\in A\land (x\in B\setminus A)\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Definition\;von\;\setminus }}\right.}\\[0.3em]=\;&\{x\,|\,x\in A\land x\in B\land x\notin A\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {die\;Konjunktion\;ist\;immer\;falsch}}\right.}\\[0.3em]=\;&\{x\,|\,F\land x\in B\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Eigenschaft\;von\;\land }}\right.}\\[0.3em]=\;&\varnothing \end{aligned}}}
Lösung Teilaufgabe 6:
A
∪
(
B
∖
A
)
↕
D
e
f
i
n
i
t
i
o
n
v
o
n
∪
=
{
x
|
x
∈
A
∨
(
x
∈
B
∖
A
)
}
↕
D
e
f
i
n
i
t
i
o
n
v
o
n
−
=
{
x
|
x
∈
A
∨
(
x
∈
B
∧
x
∉
A
}
↕
E
i
g
e
n
s
c
h
a
f
t
v
o
n
∧
=
{
x
|
(
(
x
∈
A
)
∨
(
x
∈
B
)
)
∧
(
(
x
∈
A
)
∨
(
x
∉
A
)
)
}
↕
d
i
e
D
i
s
j
u
n
k
t
i
o
n
i
s
t
i
m
m
e
r
w
a
h
r
=
{
x
|
(
(
x
∈
A
)
∨
(
x
∈
B
)
)
∧
T
}
↕
D
e
f
i
n
i
t
i
o
n
v
o
n
∪
=
A
∪
B
{\displaystyle {\begin{aligned}&A\cup (B\setminus A)\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Definition\;von\;\cup }}\right.}\\[0.3em]=\;&\{x\,|\,x\in A\lor (x\in B\setminus A)\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Definition\;von\;-}}\right.}\\[0.3em]=\;&\{x\,|\,x\in A\lor (x\in B\land x\notin A\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Eigenschaft\;von\;\land }}\right.}\\[0.3em]=\;&\{x\,|\,((x\in A)\lor (x\in B))\land ((x\in A)\lor (x\notin A))\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {die\;Disjunktion\;ist\;immer\;wahr}}\right.}\\[0.3em]=\;&\{x\,|\,((x\in A)\lor (x\in B))\land T\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Definition\;von\;\cup }}\right.}\\[0.3em]=\;&A\cup B\end{aligned}}}
Aufgabe (Große unendliche Vereinigung und Durchschnitt)
Seien die Mengen
A
i
=
{
1
,
2
,
3
,
.
.
.
,
i
}
{\displaystyle A_{i}=\{1,2,3,...,i\}}
i
=
1
,
2
,
3
,
.
.
.
{\displaystyle i=1,2,3,...}
⋃
i
=
1
n
A
i
{\displaystyle \bigcup \limits _{i=1}^{n}A_{i}}
Wie kommt man auf den Beweis? (Große unendliche Vereinigung und Durchschnitt)
Beachte, dass jeder Wert
i
=
1
,
2
,
3
,
.
.
.
{\displaystyle i=1,2,3,...}
A
i
=
{
1
,
2
,
3
,
.
.
.
,
i
}
{\displaystyle A_{i}=\{1,2,3,...,i\}}
i
=
1
{\displaystyle i=1}
A
1
=
{
1
}
{\displaystyle A_{1}=\{1\}}
i
=
2
{\displaystyle i=2}
A
2
=
{
1
,
2
}
{\displaystyle A_{2}=\{1,2\}}
i
=
3
{\displaystyle i=3}
A
3
=
{
1
,
2
,
3
}
{\displaystyle A_{3}=\{1,2,3\}}
⋃
i
=
1
n
A
i
=
A
1
∪
A
2
∪
A
3
∪
.
.
.
∪
A
n
{\displaystyle \bigcup \limits _{i=1}^{n}A_{i}=A_{1}\cup A_{2}\cup A_{3}\cup ...\cup A_{n}}
Lösung (Große unendliche Vereinigung und Durchschnitt)
⋃
i
=
1
n
A
i
=
{
1
,
2
,
3
,
.
.
.
,
n
}
{\displaystyle \bigcup \limits _{i=1}^{n}A_{i}=\{1,2,3,...,n\}}
Lösung (Eigenschaften der symmetrischen Differenz)
Lösung Teilaufgabe 1:
A
△
A
=
(
A
∖
A
)
∪
(
A
∖
A
)
=
∅
∪
∅
=
∅
{\displaystyle A\triangle A=(A\setminus A)\cup (A\setminus A)=\varnothing \cup \varnothing =\varnothing }
Lösung Teilaufgabe 2:
A
△
∅
=
(
A
∖
∅
)
∪
(
∅
∖
A
)
=
A
∪
∅
=
A
{\displaystyle A\triangle \varnothing =(A\setminus \varnothing )\cup (\varnothing \setminus A)=A\cup \varnothing =A}
Lösung Teilaufgabe 3:
A
△
U
=
(
A
∖
U
)
∪
(
U
∖
A
)
=
∅
∪
A
∁
=
A
∁
{\displaystyle A\triangle U=(A\setminus U)\cup (U\setminus A)=\varnothing \cup A^{\complement }=A^{\complement }}
Lösung Teilaufgabe 4:
A
△
A
∁
=
(
A
∖
A
∁
)
∪
(
A
∁
∖
A
)
=
A
∪
A
∁
=
U
{\displaystyle A\triangle A^{\complement }=(A\setminus A^{\complement })\cup (A^{\complement }\setminus A)=A\cup A^{\complement }=U}
Verständnisfrage: Seien die Mengen
A
i
=
{
1
,
2
,
3
,
.
.
.
,
i
}
{\displaystyle A_{i}=\{1,2,3,...,i\}}
i
=
1
,
2
,
3
,
.
.
.
{\displaystyle i=1,2,3,...}
⋃
i
=
1
∞
A
i
{\displaystyle \bigcup \limits _{i=1}^{\infty }A_{i}}
⋂
i
=
1
∞
A
i
{\displaystyle \bigcap \limits _{i=1}^{\infty }A_{i}}
Beachte, dass jeder Wert
i
=
1
,
2
,
3
,
.
.
.
{\displaystyle i=1,2,3,...}
A
i
=
{
1
,
2
,
3
,
.
.
.
,
i
}
{\displaystyle A_{i}=\{1,2,3,...,i\}}
i
=
1
{\displaystyle i=1}
A
1
=
{
1
}
{\displaystyle A_{1}=\{1\}}
i
=
2
{\displaystyle i=2}
A
2
=
{
1
,
2
}
{\displaystyle A_{2}=\{1,2\}}
i
=
3
{\displaystyle i=3}
A
3
=
{
1
,
2
,
3
}
{\displaystyle A_{3}=\{1,2,3\}}
⋃
i
=
1
∞
A
i
=
A
1
∪
A
2
∪
A
3
∪
.
.
.
{\displaystyle \bigcup \limits _{i=1}^{\infty }A_{i}=A_{1}\cup A_{2}\cup A_{3}\cup ...}
A
i
{\displaystyle A_{i}}
⋂
i
=
1
∞
A
i
=
A
1
∩
A
2
∩
A
3
∩
.
.
.
{\displaystyle \bigcap \limits _{i=1}^{\infty }A_{i}=A_{1}\cap A_{2}\cap A_{3}\cap ...}
Dann die unendliche Vereinigung und Durchschnitt in der Reihenfolge sind:
⋃
i
=
1
∞
A
i
=
{
1
,
2
,
3
,
.
.
.
}
=
Z
+
{\displaystyle \bigcup \limits _{i=1}^{\infty }A_{i}=\{1,2,3,...\}=\mathbb {Z} ^{+}}
⋂
i
=
1
∞
A
i
=
{
1
}
{\displaystyle \bigcap \limits _{i=1}^{\infty }A_{i}=\{1\}}


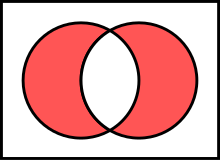





























![{\displaystyle {\begin{aligned}A\,\triangle \,B&:=(A\setminus B)\cup (B\setminus A)\\[0.3em]&=\{x\in A\cup B|\,(x\in A\land x\notin B)\lor (x\notin A\land x\in B)\}\\[0.3em]&=\{x\in A\cup B|x\in A\,{\dot {\lor }}\,x\in B\}\\[0.3em]\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/12d66b766ce3a0e8c79a9b2e42d4a9a2744e973e)












![{\displaystyle {\begin{aligned}&A\cup A^{\complement }\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Definition\;von\;^{\complement }}}\right.}\\[0.3em]=\;&A\cup \{y|\,y\notin A\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Definition\;von\;\cup }}\right.}\\[0.3em]=\;&\{x\;|\;x\in A\lor x\in \{y|\,y\notin A\}\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Abstraktionsprinzip\;f{\ddot {u}}r\;x\notin \{y\,|\,y\notin A\}}}\right.}\\[0.3em]=\;&\{x\;|\;x\in A\lor x\notin A\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {die\;Disjunktion\;ist\;immer\;wahr}}\right.}\\[0.3em]=\;&\{x\;|\;x=x\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Definition\;von\;{\mathcal {V}}}}\right.}\\[0.3em]=\;&{\mathcal {V}}\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/03717020c4ed8c23671e56620427ffc5e2f83b38)
![{\displaystyle {\begin{aligned}&(A\cup B)^{\complement }\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Definition\;von\;^{\complement }}}\right.}\\[0.3em]=\;&\{x\;|\;x\notin A\cup B\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Definition\;von\;\cup }}\right.}\\[0.3em]=\;&\{x\;|\;x\notin \{y\;|\;y\in A\lor y\in B\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Abstraktionsprinzip\;f{\ddot {u}}r\;x\notin \{y\,|\,y\in A\lor y\in B\}}}\right.}\\[0.3em]=\;&\{x\;|\;\neg \,(x\in A\lor x\in B)\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {de\;Morgansche\;Regel\;f{\ddot {u}}r\;\neg \;und\;\lor }}\right.}\\[0.3em]=\;&\{x\;|\;x\notin A\land x\notin B\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Abstraktionsprinzip\;f{\ddot {u}}r\;x\notin A\;und\;x\notin B}}\right.}\\[0.3em]=\;&\{x\;|\;x\in \{y\,|y\notin A\}\land x\in \{z\,|\,z\notin B\}\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Definition\;von\;\complement }}\right.}\\[0.3em]=\;&\{x\;|\;x\in A^{\complement }\land x\in B^{\complement }\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Definition\;von\;\cap }}\right.}\\[0.3em]=\;&A^{\complement }\cap B^{\complement }\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/d48b7b3d18e3872976d25f66205359a81098165d)
![{\displaystyle {\begin{aligned}&\varnothing ^{\complement }\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Definition\;von\;^{\complement }}}\right.}\\[0.3em]=\;&\{x\,|\,x\notin \varnothing \}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ x\notin \varnothing \;{\mathsf {ist\;immer\;wahr}}\right.}\\[0.3em]=\;&\{x\,|\,x=x\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Definition\;von\;{\mathcal {V}}}}\right.}\\[0.3em]=\;&{\mathcal {V}}\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/597cf03a7c298060b5f34221596869b92613e951)
![{\displaystyle {\begin{aligned}&(A\cap B)\cup (A\cap B^{\complement })\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Definition\;von\;\cup }}\right.}\\[0.3em]=\;&\{x\,|\,x\in (A\cap B)\,\lor \,x\in (A\cap B^{\complement })\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Definition\;von\;\cap }}\right.}\\[0.3em]=\;&\{x\,|\,((x\in A)\land (x\in B))\,\lor \,((x\in A)\land (x\in B^{\complement }))\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Distributivgesetz\;von\;\land \;an\;\lor }}\right.}\\[0.3em]=\;&\{x\,|\,(x\in A)\land ((x\in B)\,\lor \,(x\in B^{\complement }))\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Eigenschaft\;von\;\lor }}\right.}\\[0.3em]=\;&\{x\,|\,(x\in A)\land T\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Eigenschaft\;von\;\land }}\right.}\\[0.3em]=\;&\{x\,|\,(x\in A)\}\\[0.3em]=\;&A\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/64df2d67193507573f94d4ed29b4a4d212c6cade)
![{\displaystyle {\begin{aligned}&A\cap (B\setminus A)\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Definition\;von\;\cap }}\right.}\\[0.3em]=\;&\{x\,|\,x\in A\land (x\in B\setminus A)\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Definition\;von\;\setminus }}\right.}\\[0.3em]=\;&\{x\,|\,x\in A\land x\in B\land x\notin A\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {die\;Konjunktion\;ist\;immer\;falsch}}\right.}\\[0.3em]=\;&\{x\,|\,F\land x\in B\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Eigenschaft\;von\;\land }}\right.}\\[0.3em]=\;&\varnothing \end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/2a6ae40bcaaf53e27617ed30a7d73bb74c97dfc1)
![{\displaystyle {\begin{aligned}&A\cup (B\setminus A)\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Definition\;von\;\cup }}\right.}\\[0.3em]=\;&\{x\,|\,x\in A\lor (x\in B\setminus A)\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Definition\;von\;-}}\right.}\\[0.3em]=\;&\{x\,|\,x\in A\lor (x\in B\land x\notin A\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Eigenschaft\;von\;\land }}\right.}\\[0.3em]=\;&\{x\,|\,((x\in A)\lor (x\in B))\land ((x\in A)\lor (x\notin A))\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {die\;Disjunktion\;ist\;immer\;wahr}}\right.}\\[0.3em]=\;&\{x\,|\,((x\in A)\lor (x\in B))\land T\}\\[0.3em]&{\color {OliveGreen}\left\updownarrow \ {\mathsf {Definition\;von\;\cup }}\right.}\\[0.3em]=\;&A\cup B\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/5e77d70d9e69d8f184a9d8c80c419329b6142ae8)

























